how to improve your problem solving skills in math

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Free ready-to-use math resources

Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

20 Effective Math Strategies To Approach Problem-Solving 

Katie Keeton

Math strategies for problem-solving help students use a range of approaches to solve many different types of problems. It involves identifying the problem and carrying out a plan of action to find the answer to mathematical problems.  

Problem-solving skills are essential to math in the general classroom and real-life. They require logical reasoning and critical thinking skills. Students must be equipped with strategies to help them find solutions to problems.

This article explores mathematical problem solving strategies, logical reasoning and critical thinking skills to help learners with solving math word problems independently in real-life situations. 

What are problem-solving strategies?

Problem-solving strategies in math are methods students can use to figure out solutions to math problems. Some problem-solving strategies: 

• Draw a model
• Use different approaches
• Check the inverse to make sure the answer is correct

Students need to have a toolkit of math problem-solving strategies at their disposal to provide different ways to approach math problems. This makes it easier to find solutions and understand math better. 

Strategies can help guide students to the solution when it is difficult ot know when to start.

The ultimate guide to problem solving techniques

Download these ready-to-go problem solving techniques that every student should know. Includes printable tasks for students including challenges, short explanations for teachers with questioning prompts.

20 Math Strategies For Problem-Solving

Different problem-solving math strategies are required for different parts of the problem. It is unlikely that students will use the same strategy to understand and solve the problem. 

Here are 20 strategies to help students develop their problem-solving skills. 

Strategies to understand the problem

Strategies that help students understand the problem before solving it helps ensure they understand: 

• The context
• What the key information is
• How to form a plan to solve it

Following these steps leads students to the correct solution and makes the math word problem easier .

Here are five strategies to help students understand the content of the problem and identify key information. 

1. Read the problem aloud

Read a word problem aloud to help understand it. Hearing the words engages auditory processing. This can make it easier to process and comprehend the context of the situation.

2. Highlight keywords 

When keywords are highlighted in a word problem, it helps the student focus on the essential information needed to solve it. Some important keywords help determine which operation is needed.  For example, if the word problem asks how many are left, the problem likely requires subtraction.  Ensure students highlight the keywords carefully and do not highlight every number or keyword. There is likely irrelevant information in the word problem.

3. Summarize the information

Read the problem aloud, highlight the key information and then summarize the information. Students can do this in their heads or write down a quick summary.  Summaries should include only the important information and be in simple terms that help contextualize the problem.

4. Determine the unknown

A common problem that students have when solving a word problem is misunderstanding what they are solving. Determine what the unknown information is before finding the answer.  Often, a word problem contains a question where you can find the unknown information you need to solve. For example, in the question ‘How many apples are left?’ students need to find the number of apples left over.

5. Make a plan

Once students understand the context of the word problem, have dentified the important information and determined the unknown, they can make a plan to solve it.  The plan will depend on the type of problem. Some problems involve more than one step to solve them as some require more than one answer.  Encourage students to make a list of each step they need to take to solve the problem before getting started.

Strategies for solving the problem 

1. draw a model or diagram.

Students may find it useful to draw a model, picture, diagram, or other visual aid to help with the problem solving process.  It can help to visualize the problem to understand the relationships between the numbers in the problem. In turn, this helps students see the solution.

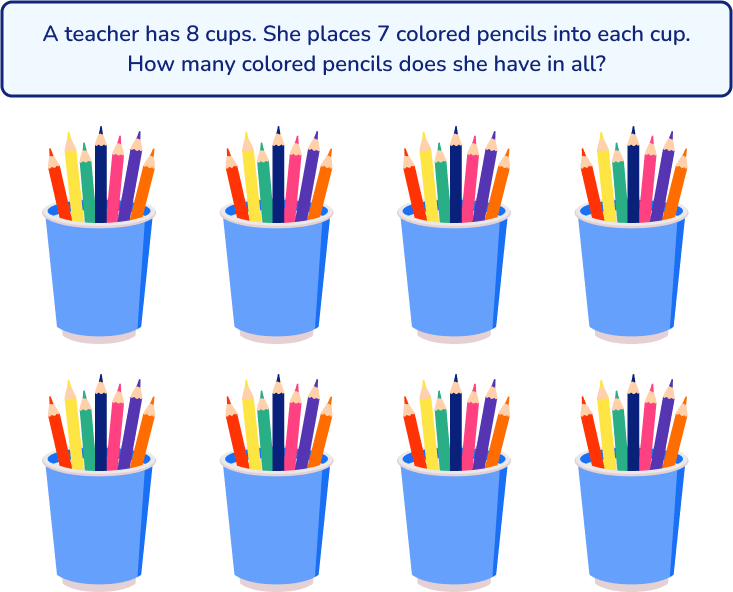
Similarly, you could draw a model to represent the objects in the problem:

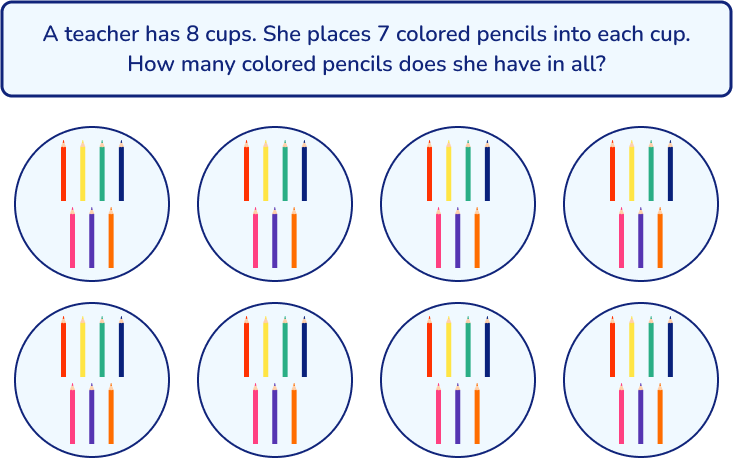
2. Act it out

This particular strategy is applicable at any grade level but is especially helpful in math investigation in elementary school . It involves a physical demonstration or students acting out the problem using movements, concrete resources and math manipulatives .  When students act out a problem, they can visualize and contectualize the word problem in another way and secure an understanding of the math concepts.  The examples below show how 1st-grade students could “act out” an addition and subtraction problem:

3. Work backwards

Working backwards is a popular problem-solving strategy. It involves starting with a possible solution and deciding what steps to take to arrive at that solution.  This strategy can be particularly helpful when students solve math word problems involving multiple steps. They can start at the end and think carefully about each step taken as opposed to jumping to the end of the problem and missing steps in between.

For example,

To solve this problem working backwards, start with the final condition, which is Sam’s grandmother’s age (71) and work backwards to find Sam’s age. Subtract 20 from the grandmother’s age, which is 71.  Then, divide the result by 3 to get Sam’s age. 71 – 20 = 51 51 ÷ 3 = 17 Sam is 17 years old.

4. Write a number sentence

When faced with a word problem, encourage students to write a number sentence based on the information. This helps translate the information in the word problem into a math equation or expression, which is more easily solved.  It is important to fully understand the context of the word problem and what students need to solve before writing an equation to represent it.

5. Use a formula

Specific formulas help solve many math problems. For example, if a problem asks students to find the area of a rug, they would use the area formula (area = length × width) to solve.   Make sure students know the important mathematical formulas they will need in tests and real-life. It can help to display these around the classroom or, for those who need more support, on students’ desks.

Strategies for checking the solution 

Once the problem is solved using an appropriate strategy, it is equally important to check the solution to ensure it is correct and makes sense. 

There are many strategies to check the solution. The strategy for a specific problem is dependent on the problem type and math content involved.

Here are five strategies to help students check their solutions. 

1. Use the Inverse Operation

For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7. As good practice, encourage students to use the inverse operation routinely to check their work. 

2. Estimate to check for reasonableness

Once students reach an answer, they can use estimation or rounding to see if the answer is reasonable.  Round each number in the equation to a number that’s close and easy to work with, usually a multiple of ten.  For example, if the question was 216 ÷ 18 and the quotient was 12, students might round 216 to 200 and round 18 to 20. Then use mental math to solve 200 ÷ 20, which is 10.  When the estimate is clear the two numbers are close. This means your answer is reasonable. 

3. Plug-In Method

This method is particularly useful for algebraic equations. Specifically when working with variables.  To use the plug-in method, students solve the problem as asked and arrive at an answer. They can then plug the answer into the original equation to see if it works. If it does, the answer is correct.

If students use the equation 20m+80=300 to solve this problem and find that m = 11, they can plug that value back into the equation to see if it is correct. 20m + 80 = 300 20 (11) + 80 = 300 220 + 80 = 300 300 = 300 ✓

4. Peer Review

Peer review is a great tool to use at any grade level as it promotes critical thinking and collaboration between students. The reviewers can look at the problem from a different view as they check to see if the problem was solved correctly.   Problem solvers receive immediate feedback and the opportunity to discuss their thinking with their peers. This strategy is effective with mixed-ability partners or similar-ability partners. In mixed-ability groups, the partner with stronger skills provides guidance and support to the partner with weaker skills, while reinforcing their own understanding of the content and communication skills.  If partners have comparable ability levels and problem-solving skills, they may find that they approach problems differently or have unique insights to offer each other about the problem-solving process.

5. Use a Calculator

A calculator can be introduced at any grade level but may be best for older students who already have a foundational understanding of basic math operations. Provide students with a calculator to allow them to check their solutions independently, accurately, and quickly. Since calculators are so readily available on smartphones and tablets, they allow students to develop practical skills that apply to real-world situations.  

Step-by-step problem-solving processes for your classroom

In his book, How to Solve It , published in 1945, mathematician George Polya introduced a 4-step process to solve problems. 

Polya’s 4 steps include:

• Understand the problem
• Devise a plan
• Carry out the plan

Today, in the style of George Polya, many problem-solving strategies use various acronyms and steps to help students recall. 

Many teachers create posters and anchor charts of their chosen process to display in their classrooms. They can be implemented in any elementary, middle school or high school classroom. 

Here are 5 problem-solving strategies to introduce to students and use in the classroom.

How Third Space Learning improves problem-solving 

Resources .

Third Space Learning offers a free resource library is filled with hundreds of high-quality resources. A team of experienced math experts carefully created each resource to develop students mental arithmetic, problem solving and critical thinking. 

Explore the range of problem solving resources for 2nd to 8th grade students. 

One-on-one tutoring 

Third Space Learning offers one-on-one math tutoring to help students improve their math skills. Highly qualified tutors deliver high-quality lessons aligned to state standards. 

Former teachers and math experts write all of Third Space Learning’s tutoring lessons. Expertly designed lessons follow a “my turn, follow me, your turn” pedagogy to help students move from guided instruction and problem-solving to independent practice. 

Throughout each lesson, tutors ask higher-level thinking questions to promote critical thinking and ensure students are developing a deep understanding of the content and problem-solving skills.

Problem-solving

Educators can use many different strategies to teach problem-solving and help students develop and carry out a plan when solving math problems. Incorporate these math strategies into any math program and use them with a variety of math concepts, from whole numbers and fractions to algebra. 

Teaching students how to choose and implement problem-solving strategies helps them develop mathematical reasoning skills and critical thinking they can apply to real-life problem-solving.

READ MORE : 8 Common Core math examples

There are many different strategies for problem-solving; Here are 5 problem-solving strategies: • draw a model  • act it out  • work backwards  • write a number sentence • use a formula

Here are 10 strategies of problem-solving: • Read the problem aloud • Highlight keywords • Summarize the information • Determine the unknown • Make a plan • Draw a model  • Act it out  • Work backwards  • Write a number sentence • Use a formula

1. Understand the problem 2. Devise a plan 3. Carry out the plan 4. Look back

Some strategies you can use to solve challenging math problems are: breaking the problem into smaller parts, using diagrams or models, applying logical reasoning, and trying different approaches.

Related articles

Why Student Centered Learning Is Important: A Guide For Educators

13 Effective Learning Strategies: A Guide to Using them in your Math Classroom

Differentiated Instruction: 9 Differentiated Curriculum And Instruction Strategies For Teachers 

5 Math Mastery Strategies To Incorporate Into Your 4th and 5th Grade Classrooms

Ultimate Guide to Metacognition [FREE]

Looking for a summary on metacognition in relation to math teaching and learning?

Check out this guide featuring practical examples, tips and strategies to successfully embed metacognition across your school to accelerate math growth.

Privacy Overview

How to Improve Problem-Solving Skills: Mathematics and Critical Thinking

In today’s rapidly changing world, problem-solving has become a quintessential skill. When we discuss the topic, it’s natural to ask, “What is problem-solving?” and “How can we enhance this skill, particularly in children?” The discipline of mathematics offers a rich platform to explore these questions. Through math, not only do we delve into numbers and equations, but we also explore how to improve problem-solving skills and how to develop critical thinking skills in math. Let’s embark on this enlightening journey together.

What is Problem-Solving?

At its core, problem-solving involves identifying a challenge and finding a solution. But it’s not always as straightforward as it sounds. So, what is problem-solving? True problem-solving requires a combination of creative thinking and logical reasoning. Mathematics, in many ways, embodies this blend. When a student approaches a math problem, they must discern the issue at hand, consider various methods to tackle it, and then systematically execute their chosen strategy.

But what is problem-solving in a broader context? It’s a life skill. Whether we’re deciding the best route to a destination, determining how to save for a big purchase, or even figuring out how to fix a broken appliance, we’re using problem-solving.

How to Develop Critical Thinking Skills in Math

Critical thinking goes hand in hand with problem-solving. But exactly how to develop critical thinking skills in math might not be immediately obvious. Here are a few strategies:

• Contextual Learning: Teaching math within a story or real-life scenario makes it relevant. When students see math as a tool to navigate the world around them, they naturally begin to think critically about solutions.
• Open-ended Questions: Instead of merely seeking the “right” answer, encourage students to explain their thought processes. This nudges them to think deeply about their approach.
• Group Discussions: Collaborative learning can foster different perspectives, prompting students to consider multiple ways to solve a problem.
• Challenging Problems: Occasionally introducing problems that are a bit beyond a student’s current skill level can stimulate critical thinking. They will have to stretch their understanding and think outside the box.

What are the Six Basic Steps of the Problem-Solving Process?

Understanding how to improve problem-solving skills often comes down to familiarizing oneself with the systematic approach to challenges. So, what are the six basic steps of the problem-solving process?

• Identification: Recognize and define the problem.
• Analysis: Understand the problem’s intricacies and nuances.
• Generation of Alternatives: Think of different ways to approach the challenge.
• Decision Making: Choose the most suitable method to address the problem.
• Implementation: Put the chosen solution into action.
• Evaluation: Reflect on the solution’s effectiveness and learn from the outcome.

By embedding these steps into mathematical education, we provide students with a structured framework. When they wonder about how to improve problem-solving skills or how to develop critical thinking skills in math, they can revert to this process, refining their approach with each new challenge.

Making Math Fun and Relevant

At Wonder Math, we believe that the key to developing robust problem-solving skills lies in making math enjoyable and pertinent. When students see math not just as numbers on a page but as a captivating story or a real-world problem to be solved, their engagement skyrockets. And with heightened engagement comes enhanced understanding.

As educators and parents, it’s crucial to continuously ask ourselves: how can we demonstrate to our children what problem-solving is? How can we best teach them how to develop critical thinking skills in math? And how can we instill in them an understanding of the six basic steps of the problem-solving process?

The answer, we believe, lies in active learning, contextual teaching, and a genuine passion for the beauty of mathematics.

The Underlying Beauty of Mathematics

Often, people perceive mathematics as a rigid discipline confined to numbers and formulas. However, this is a limited view. Math, in essence, is a language that describes patterns, relationships, and structures. It’s a medium through which we can communicate complex ideas, describe our universe, and solve intricate problems. Understanding this deeper beauty of math can further emphasize how to develop critical thinking skills in math.

Why Mathematics is the Ideal Playground for Problem-Solving

Math provides endless opportunities for problem-solving. From basic arithmetic puzzles to advanced calculus challenges, every math problem offers a chance to hone our problem-solving skills. But why is mathematics so effective in this regard?

• Structured Challenges: Mathematics presents problems in a structured manner, allowing learners to systematically break them down. This format mimics real-world scenarios where understanding the structure of a challenge can be half the battle.
• Multiple Approaches: Most math problems can be approached in various ways . This teaches learners flexibility in thinking and the ability to view a single issue from multiple angles.
• Immediate Feedback: Unlike many real-world problems where solutions might take time to show results, in math, students often get immediate feedback. They can quickly gauge if their approach works or if they need to rethink their strategy.

Enhancing the Learning Environment

To genuinely harness the power of mathematics in developing problem-solving skills, the learning environment plays a crucial role. A student who is afraid of making mistakes will hesitate to try out different approaches, stunting their critical thinking growth.

However, in a nurturing, supportive environment where mistakes are seen as learning opportunities, students thrive. They become more willing to take risks, try unconventional solutions, and learn from missteps. This mindset, where failure is not feared but embraced as a part of the learning journey, is pivotal for developing robust problem-solving skills.

Incorporating Technology

In our digital age, technology offers innovative ways to explore math. Interactive apps and online platforms can provide dynamic problem-solving scenarios, making the process even more engaging. These tools can simulate real-world challenges, allowing students to apply their math skills in diverse contexts, further answering the question of how to improve problem-solving skills.

More than Numbers 

In summary, mathematics is more than just numbers and formulas—it’s a world filled with challenges, patterns, and beauty. By understanding its depth and leveraging its structured nature, we can provide learners with the perfect platform to develop critical thinking and problem-solving skills. The key lies in blending traditional techniques with modern tools, creating a holistic learning environment that fosters growth, curiosity, and a lifelong love for learning.

Join us on this transformative journey at Wonder Math. Let’s make math an adventure, teaching our children not just numbers and equations, but also how to improve problem-solving skills and navigate the world with confidence. Enroll your child today and witness the magic of mathematics unfold before your eyes!

FAQ: Mathematics and Critical Thinking

1. what is problem-solving in the context of mathematics.

Problem-solving in mathematics refers to the process of identifying a mathematical challenge and systematically working through methods and strategies to find a solution.

2. Why is math considered a good avenue for developing problem-solving skills?

Mathematics provides structured challenges and allows for multiple approaches to find solutions. This promotes flexibility in thinking and encourages learners to view problems from various angles.

3. How does contextual learning enhance problem-solving abilities?

By teaching math within a story or real-life scenario, it becomes more relevant for the learner. This helps them see math as a tool to navigate real-world challenges , thereby promoting critical thinking.

4. What are the six basic steps of the problem-solving process in math?

The six steps are: Identification, Analysis, Generation of Alternatives, Decision Making, Implementation, and Evaluation.

5. How can parents support their children in developing mathematical problem-solving skills?

Parents can provide real-life contexts for math problems , encourage open discussions about different methods, and ensure a supportive environment where mistakes are seen as learning opportunities.

6. Are there any tools or apps that can help in enhancing problem-solving skills in math?

Yes, there are various interactive apps and online platforms designed specifically for math learning. These tools provide dynamic problem-solving scenarios and simulate real-world challenges, making the learning process engaging.

7. How does group discussion foster critical thinking in math?

Group discussions allow students to hear different perspectives and approaches to a problem. This can challenge their own understanding and push them to think about alternative methods.

8. Is it necessary to always follow the six steps of the problem-solving process sequentially?

While the six steps provide a structured approach, real-life problem-solving can sometimes be more fluid. It’s beneficial to know the steps, but adaptability and responsiveness to the situation are also crucial.

9. How does Wonder Math incorporate active learning in teaching mathematics?

Wonder Math integrates mathematics within engaging stories and real-world scenarios, making it fun and relevant. This active learning approach ensures that students are not just passive recipients but active participants in the learning process.

10. What if my child finds a math problem too challenging and becomes demotivated?

It’s essential to create a supportive environment where challenges are seen as growth opportunities. Remind them that every problem is a chance to learn, and it’s okay to seek help or approach it differently.

Related posts

Summer Math Programs: How They Can Prevent Learning Loss in Young Students

As summer approaches, parents and educators alike turn their attention to how they can support young learners during the break. Summer is a time for relaxation, fun, and travel, yet it’s also a critical period when learning loss can occur. This phenomenon, often referred to as the “summer slide,” impacts students’ progress, especially in foundational subjects like mathematics. It’s reported…

Math Programs 101: What Every Parent Should Know When Looking For A Math Program

  As a parent, you know that a solid foundation in mathematics is crucial for your child’s success, both in school and in life. But with so many math programs and math help services out there, how do you choose the right one? Whether you’re considering Outschool classes, searching for “math tutoring near me,” or exploring tutoring services online, understanding…

• Prodigy Math
• Prodigy English
• Is a Premium Membership Worth It?
• Promote a Growth Mindset
• Help Your Child Who's Struggling with Math
• Parent's Guide to Prodigy
• Assessments
• Math Curriculum Coverage
• English Curriculum Coverage
• Game Portal

9 Ways to Improve Math Skills Quickly & Effectively

Written by Ashley Crowe

Help your child improve their math skills with the game that makes learning an adventure!

• Parent Resources

The importance of understanding basic math skills

• 9 Ways to improve math skills
• How to use technology to improve math skills

Math class can move pretty fast. There’s so much to cover in the course of a school year. And if your child doesn’t get a new math idea right away, they can quickly get left behind.

If your child is struggling with basic math problems every day, it doesn’t mean they’re destined to be bad at math. Some students need more time to develop the problem-solving skills that math requires. Others may need to revisit past concepts before moving on. Because of how math is structured, it’s best to take each year step-by-step, lesson by lesson.

This article has tips and tricks to improve your child’s math skills while minimizing frustrations and struggles. If your child is growing to hate math, read on for ways to improve their skills and confidence, and maybe even make math fun! 

But first, the basics.

Math is a subject that builds on itself. It takes a solid understanding of past concepts to prepare for the next lesson. 

That’s why math can become frustrating when you’re forced to move on before you’re ready. You’re either stuck trying to catch up or you end up falling further behind.

But with a strong understanding of basic math skills, your child can be set up for school success. If you’re unfamiliar with the idea of sets or whole numbers , this is a great place to start. 

What are considered basic math skills?

The basic math skills required to move on to higher levels of math learning are: 

• Addition — Adding to a set.
• Subtraction — Taking away from a set.
• Multiplication — Adding equal sets together in groups (2 sets of 3 is the same as 2x3, or 6).
• Division — How many equal sets can be found in a number (12 has how many sets of two in it? 6 sets of 2).
• Percentages — A specific amount in relation to 100.
• Fractions & Decimals — Fractions are equal parts of a whole set. Decimals represent a number of parts of a whole in relation to 10. These both contrast with whole numbers. 
• Spatial Reasoning — How numbers and shapes fit together.

How to improve math skills 

People aren’t bad at math — many just need more time and practice to gain a thorough understanding.

How can you help your child improve their math abilities? Use our top 9 tips for quickly and effectively improving math skills .

1. Wrap your head around the concepts

Repetition and practice are great, but if you don’t understand the concept , it will be difficult to move forward. 

Luckily, there are many great ways to break down math concepts . The trick is finding the one that works best for your child.

Math manipulatives can be a game-changer for children who are struggling with big math ideas. Taking math off the page and putting it into their hands can bring ideas to life. Numbers become less abstract and more concrete when you’re counting toy cars or playing with blocks. Creating these “sets” of objects can bring clarity to basic math learning.

2. Try game-based learning

During math practice, repetition is important — but it can get old in a hurry. No one enjoys copying their times tables over and over and over again. If learning math has become a chore, it’s time to bring back the fun! 

Game-based learning is a great way to practice new concepts and solidify past lessons. It can even make repetition fun and engaging.

Game-based learning can look like a family board game on Friday night or an educational app , like Prodigy Math .

Take math from frustrating to fun with the right game, then watch the learning happen easily!

3. Bring math into daily life

You use basic math every day. 

As you go about your day, help your child see the math that’s all around them:  

• Tell them how fast you’re driving on the way to school
• Calculate the discount you’ll receive on your next Target trip
• Count out the number of apples you need to buy at the grocery store
• While baking, explain how 6 quarter cups is the same amount of flour as a cup and a half — then enjoy some cookies!

Relate math back to what your child loves and show them how it’s used every day. Math doesn’t have to be mysterious or abstract. Instead, use math to race monster trucks or arrange tea parties. Break it down, take away the fear, and watch their interest in math grow.

4. Implement daily practice

Math practice is important. Once you understand the concept, you have to nail down the mechanics. And often, it’s the practice that finally helps the concept click. Either way, math requires more than just reading formulas on a page.

Daily practice can be tough to implement, especially with a math-averse child. This is a great time to bring out the game-based learning mentioned above. Or find an activity that lines up with their current lesson. Are they learning about squares? Break out the math link cubes and create them. Whenever possible, step away from the worksheets and flashcards and find practice elsewhere.

5. Sketch word problems

Nothing causes a panic quite like an unexpected word problem. Something about the combination of numbers and words can cause the brain of a struggling math learner to shut down. But it doesn’t have to be that way.

Many word problems just need to be broken down, step by step . One great way to do this is to sketch it out. If Doug has five apples and four oranges, then eats two of each, how many does he have left? Draw it, talk it out, cross them off, then count. 

If you’ve been talking your child through the various math challenges you encounter every day, many word problems will start to feel familiar. 

6. Set realistic goals

If your child has fallen behind in math, then more study time is the answer. But forcing them to cram an extra hour of math in their day is not likely to produce better results. To see a positive change, first identify their biggest struggles . Then set realistic goals addressing these issues . 

Two more hours of practicing a concept they don’t understand is only going to cause more frustration. Even if they can work through the mechanics of a problem, the next lesson will leave them feeling just as lost. 

Instead, try mini practice sessions and enlist some extra help. Approach the problem in a new way, reach out to their teacher or try an online math lesson . Make sure the extra time is troubleshooting the actual problem, not just reinforcing the idea that math is hard and no fun. 

Set Goals and Rewards in Prodigy Math

Did you know that parents can set learning goals for their child in Prodigy Math? And once they achieve them, they'll unlock in-game rewards of your choice!

7. Engage with a math tutor

If your child is struggling with big picture concepts, look into finding a math tutor . Everyone learns differently, and you and your child’s teacher may be missing that “aha” moment that a little extra time and the right tutor can provide.

It’s amazing when a piece of the math puzzle finally clicks for your child. If you’re ready to get that extra help, try a free 1:1 online session from Prodigy Math Tutoring. Prodigy’s tutors are real teachers who know how to connect kids to math. With the right approach, your child can become confident in math — and who knows, they may even begin to enjoy it. 

8. Focus on one concept at a time

Math builds on itself. If your child is struggling through their current lesson, they can’t skip it and come back to it later. This is the time to practice and repeat — re-examining and reinforcing the current concept until it makes sense.

Look for other ways to approach new math ideas. Use math manipulatives to bring numbers off the page. Or try a learning app with exciting rewards and positive reinforcement to encourage extra practice. 

Take a step back when frustrations get high — but resist the temptation to just let it go. Once the concept clicks, they’ll be excited to forge ahead.

9. Teach others math you already know

Even if your child is struggling in math, they’ve still learned so much since last year. Focus on the improvements they’ve made and let them showcase their knowledge. If they have younger siblings, your older child can demonstrate addition or show them how to use a number line. This is a great way to build their confidence and encourage them to keep going.

Or let them teach you how they solve new problems. Have your child talk you through the process while you solve a long division problem . You’re likely to find yourself a little rusty on the details. Play it up and get a little silly. They’ll love teaching you the ropes of this “new math.”

Embracing technology to improve math skills

Though much of your math learning was done with pencil to paper, there are many more ways to build number skills in today’s tech world. 

Your child can take live, online math courses to work through tough concepts. Or play a variety of online games, solving math puzzles and getting consistent practice while having fun.

These technical advances can help every child learn math, no matter their preferred learning or study style. If your child is a visual learner, there’s an app for that. Do they process best while working in groups? Jump online and find one. Don’t keep repeating the same lessons from their math class over and over. Branch out, try something new and watch the learning click. 

Look online for more math help

There are so many online resources, it can be hard to know where to start. 

At Prodigy, we’re happy to help you get the ball rolling on your child’s math learning, from kindergarten through 8th grade. It’s free to sign up, fun to play and exciting to watch as your child’s math understanding grows.

Sign up for a free parent account and get instant data on your child’s progress as they build more math skills with Prodigy Math Game . It’s time to take the math struggle out of your home and enjoy learning together!

• Skip to main content
• Skip to primary sidebar
• Skip to footer

Additional menu

Khan Academy Blog

Unlocking the Power of Math Learning: Strategies and Tools for Success

posted on September 20, 2023

Mathematics, the foundation of all sciences and technology, plays a fundamental role in our everyday lives. Yet many students find the subject challenging, causing them to shy away from it altogether. This reluctance is often due to a lack of confidence, a misunderstanding of unclear concepts, a move ahead to more advanced skills before they are ready, and ineffective learning methods. However, with the right approach, math learning can be both rewarding and empowering. This post will explore different approaches to learning math, strategies for success, and cutting-edge tools to help you achieve your goals.

Math Learning

Math learning can take many forms, including traditional classroom instruction, online courses, and self-directed learning. A multifaceted approach to math learning can improve understanding, engage students, and promote subject mastery. A 2014 study by the National Council of Teachers of Mathematics found that the use of multiple representations, such as visual aids, graphs, and real-world examples, supports the development of mathematical connections, reasoning, and problem-solving skills.

Moreover, the importance of math learning goes beyond solving equations and formulas. Advanced math skills are essential for success in many fields, including science, engineering, finance, health care, and technology. In fact, a report by Burning Glass Technologies found that 71% of high-salary, entry-level positions require advanced math skills.

Benefits of Math Learning

In today’s 21st-century world, having a broad knowledge base and strong reading and math skills is essential. Mathematical literacy plays a crucial role in this success. It empowers individuals to comprehend the world around them and make well-informed decisions based on data-driven understanding. More than just earning good grades in math, mathematical literacy is a vital life skill that can open doors to economic opportunities, improve financial management, and foster critical thinking. We’re not the only ones who say so:

• Math learning enhances problem-solving skills, critical thinking, and logical reasoning abilities. (Source: National Council of Teachers of Mathematics )
• It improves analytical skills that can be applied in various real-life situations, such as budgeting or analyzing data. (Source: Southern New Hampshire University )
• Math learning promotes creativity and innovation by fostering a deep understanding of patterns and relationships. (Source: Purdue University )
• It provides a strong foundation for careers in fields such as engineering, finance, computer science, and more. These careers generally correlate to high wages. (Source: U.S. Bureau of Labor Statistics )
• Math skills are transferable and can be applied across different academic disciplines. (Source: Sydney School of Education and Social Work )

How to Know What Math You Need to Learn

Often students will find gaps in their math knowledge; this can occur at any age or skill level. As math learning is generally iterative, a solid foundation and understanding of the math skills that preceded current learning are key to success. The solution to these gaps is called mastery learning, the philosophy that underpins Khan Academy’s approach to education .

Mastery learning is an educational philosophy that emphasizes the importance of a student fully understanding a concept before moving on to the next one. Rather than rushing students through a curriculum, mastery learning asks educators to ensure that learners have “mastered” a topic or skill, showing a high level of proficiency and understanding, before progressing. This approach is rooted in the belief that all students can learn given the appropriate learning conditions and enough time, making it a markedly student-centered method. It promotes thoroughness over speed and encourages individualized learning paths, thus catering to the unique learning needs of each student.

Students will encounter mastery learning passively as they go through Khan Academy coursework, as our platform identifies gaps and systematically adjusts to support student learning outcomes. More details can be found in our Educators Hub . 

Try Our Free Confidence Boosters

How to learn math.

Learning at School

One of the most common methods of math instruction is classroom learning. In-class instruction provides students with real-time feedback, practical application, and a peer-learning environment. Teachers can personalize instruction by assessing students’ strengths and weaknesses, providing remediation when necessary, and offering advanced instruction to students who need it.

Learning at Home

Supplemental learning at home can complement traditional classroom instruction. For example, using online resources that provide additional practice opportunities, interactive games, and demonstrations, can help students consolidate learning outside of class. E-learning has become increasingly popular, with a wealth of online resources available to learners of all ages. The benefits of online learning include flexibility, customization, and the ability to work at one’s own pace. One excellent online learning platform is Khan Academy, which offers free video tutorials, interactive practice exercises, and a wealth of resources across a range of mathematical topics.

Moreover, parents can encourage and monitor progress, answer questions, and demonstrate practical applications of math in everyday life. For example, when at the grocery store, parents can ask their children to help calculate the price per ounce of two items to discover which one is the better deal. Cooking and baking with your children also provides a lot of opportunities to use math skills, like dividing a recipe in half or doubling the ingredients. 

Learning Math with the Help of Artificial Intelligence (AI) 

AI-powered tools are changing the way students learn math. Personalized feedback and adaptive practice help target individual needs. Virtual tutors offer real-time help with math concepts while AI algorithms identify areas for improvement. Custom math problems provide tailored practice, and natural language processing allows for instant question-and-answer sessions. 

Using Khan Academy’s AI Tutor, Khanmigo

Transform your child’s grasp of mathematics with Khanmigo , the 24/7 AI-powered tutor that specializes in tailored, one-on-one math instruction. Available at any time, Khanmigo provides personalized support that goes beyond mere answers to nurture genuine mathematical understanding and critical thinking. Khanmigo can track progress, identify strengths and weaknesses, and offer real-time feedback to help students stay on the right track. Within a secure and ethical AI framework, your child can tackle everything from basic arithmetic to complex calculus, all while you maintain oversight using robust parental controls.

Get Math Help with Khanmigo Right Now

You can learn anything .

Math learning is essential for success in the modern world, and with the right approach, it can also be enjoyable and rewarding. Learning math requires curiosity, diligence, and the ability to connect abstract concepts with real-world applications. Strategies for effective math learning include a multifaceted approach, including classroom instruction, online courses, homework, tutoring, and personalized AI support. 

So, don’t let math anxiety hold you back; take advantage of available resources and technology to enhance your knowledge base and enjoy the benefits of math learning.

National Council of Teachers of Mathematics, “Principles to Actions: Ensuring Mathematical Success for All” , April 2014

Project Lead The Way Research Report, “The Power of Transportable Skills: Assessing the Demand and Value of the Skills of the Future” , 2020

Page. M, “Why Develop Quantitative and Qualitative Data Analysis Skills?” , 2016

Mann. EL, Creativity: The Essence of Mathematics, Journal for the Education of the Gifted. Vol. 30, No. 2, 2006, pp. 236–260, http://www.prufrock.com ’

Nakakoji Y, Wilson R.” Interdisciplinary Learning in Mathematics and Science: Transfer of Learning for 21st Century Problem Solving at University ”. J Intell. 2020 Sep 1;8(3):32. doi: 10.3390/jintelligence8030032. PMID: 32882908; PMCID: PMC7555771.

Get Khanmigo

The best way to learn and teach with AI is here. Ace the school year with our AI-powered guide, Khanmigo. 

For learners     For teachers     For parents

• Our Mission

6 Tips for Teaching Math Problem-Solving Skills

Solving word problems is tougher than computing with numbers, but elementary teachers can guide students to do the deep thinking involved.

A growing concern with students is the ability to problem-solve, especially with complex, multistep problems. Data shows that students struggle more when solving word problems than they do with computation , and so problem-solving should be considered separately from computation. Why?

Consider this. When we’re on the way to a new destination and we plug in our location to a map on our phone, it tells us what lane to be in and takes us around any detours or collisions, sometimes even buzzing our watch to remind us to turn. When I experience this as a driver, I don’t have to do the thinking. I can think about what I’m going to cook for dinner, not paying much attention to my surroundings other than to follow those directions. If I were to be asked to go there again, I wouldn’t be able to remember, and I would again seek help.

If we can switch to giving students strategies that require them to think instead of giving them too much support throughout the journey to the answer, we may be able to give them the ability to learn the skills to read a map and have several ways to get there.

Here are six ways we can start letting students do this thinking so that they can go through rigorous problem-solving again and again, paving their own way to the solution. 

1. Link problem-solving to reading

When we can remind students that they already have many comprehension skills and strategies they can easily use in math problem-solving, it can ease the anxiety surrounding the math problem. For example, providing them with strategies to practice, such as visualizing, acting out the problem with math tools like counters or base 10 blocks, drawing a quick sketch of the problem, retelling the story in their own words, etc., can really help them to utilize the skills they already have to make the task less daunting.

We can break these skills into specific short lessons so students have a bank of strategies to try on their own. Here's an example of an anchor chart that they can use for visualizing . Breaking up comprehension into specific skills can increase student independence and help teachers to be much more targeted in their problem-solving instruction. This allows students to build confidence and break down the barriers between reading and math to see they already have so many strengths that are transferable to all problems.

2. Avoid boxing students into choosing a specific operation

It can be so tempting to tell students to look for certain words that might mean a certain operation. This might even be thoroughly successful in kindergarten and first grade, but just like when our map tells us where to go, that limits students from becoming deep thinkers. It also expires once they get into the upper grades, where those words could be in a problem multiple times, creating more confusion when students are trying to follow a rule that may not exist in every problem.

We can encourage a variety of ways to solve problems instead of choosing the operation first. In first grade, a problem might say, “Joceline has 13 stuffed animals and Jordan has 17. How many more does Jordan have?” Some students might choose to subtract, but a lot of students might just count to find the amount in between. If we tell them that “how many more” means to subtract, we’re taking the thinking out of the problem altogether, allowing them to go on autopilot without truly solving the problem or using their comprehension skills to visualize it. 

3. Revisit ‘representation’

The word “representation” can be misleading. It seems like something to do after the process of solving. When students think they have to go straight to solving, they may not realize that they need a step in between to be able to support their understanding of what’s actually happening in the problem first.

Using an anchor chart like one of these ( lower grade , upper grade ) can help students to choose a representation that most closely matches what they’re visualizing in their mind. Once they sketch it out, it can give them a clearer picture of different ways they could solve the problem.

Think about this problem: “Varush went on a trip with his family to his grandmother’s house. It was 710 miles away. On the way there, three people took turns driving. His mom drove 214 miles. His dad drove 358 miles. His older sister drove the rest. How many miles did his sister drive?”

If we were to show this student the anchor chart, they would probably choose a number line or a strip diagram to help them understand what’s happening.

If we tell students they must always draw base 10 blocks in a place value chart, that doesn’t necessarily match the concept of this problem. When we ask students to match our way of thinking, we rob them of critical thinking practice and sometimes confuse them in the process. 

4. Give time to process

Sometimes as educators, we can feel rushed to get to everyone and everything that’s required. When solving a complex problem, students need time to just sit with a problem and wrestle with it, maybe even leaving it and coming back to it after a period of time.

This might mean we need to give them fewer problems but go deeper with those problems we give them. We can also speed up processing time when we allow for collaboration and talk time with peers on problem-solving tasks. 

5. Ask questions that let Students do the thinking

Questions or prompts during problem-solving should be very open-ended to promote thinking. Telling a student to reread the problem or to think about what tools or resources would help them solve it is a way to get them to try something new but not take over their thinking.

These skills are also transferable across content, and students will be reminded, “Good readers and mathematicians reread.” 

6. Spiral concepts so students frequently use problem-solving skills

When students don’t have to switch gears in between concepts, they’re not truly using deep problem-solving skills. They already kind of know what operation it might be or that it’s something they have at the forefront of their mind from recent learning. Being intentional within their learning stations and assessments about having a variety of rigorous problem-solving skills will refine their critical thinking abilities while building more and more resilience throughout the school year as they retain content learning in the process. 

Problem-solving skills are so abstract, and it can be tough to pinpoint exactly what students need. Sometimes we have to go slow to go fast. Slowing down and helping students have tools when they get stuck and enabling them to be critical thinkers will prepare them for life and allow them multiple ways to get to their own destination.

• Grade 1 Lessons
• Grade 2 Lessons
• Grade 3 Lessons
• Grade 4 Lessons
• Grade 5 Lessons
• Math Activities

How to Improve Problem-Solving Skills in Math

Importance of Problem-Solving Skills in Math

Problem-solving skills are crucial in math education , enabling students to apply mathematical concepts and principles to real-world situations. Here’s why problem-solving skills are essential in math education:

1. Application of knowledge: Problem-solving in math requires encouraging students to apply the knowledge they acquire in the classroom to tackle real-life problems. It helps them understand the relevance of math in everyday life and enhances their critical thinking skills.

2. Developing critical thinking:  Problem-solving requires students to analyze, evaluate, and think critically about different approaches and strategies to solve a problem. It strengthens their mathematical abilities and improves their overall critical thinking skills.

3. Enhancing problem-solving skills:  Math problems often have multiple solutions, encouraging students to think creatively and explore different problem-solving strategies. It helps develop their problem-solving skills, which are valuable in various aspects of life beyond math.

4. Fostering perseverance:  Problem-solving in math often requires persistence and resilience. Students must be willing to try different approaches, learn from their mistakes, and keep trying until they find a solution. It fosters a growth mindset and teaches them the value of perseverance.

Benefits of strong problem-solving skills

Having strong problem-solving skills in math offers numerous benefits for students:

1. Improved academic performance:  Students with strong problem-solving skills are likelier to excel in math and other subjects that rely on logical reasoning and critical thinking.

2. Enhanced problem-solving abilities:  Strong problem-solving skills extend beyond math and can be applied to various real-life situations. It includes decision-making, analytical thinking, and solving complex problems creatively.

3. Increased confidence:  Successfully solving math problems boosts students’ self-confidence and encourages them to tackle more challenging tasks. This confidence spills over into other areas of their academic and personal lives.

4. Preparation for future careers:  Problem-solving skills are highly sought after by employers in various fields. Developing strong problem-solving skills in math sets students up for successful careers in engineering, technology, finance, and more.

Problem-solving skills are essential for math education and have numerous benefits for students. By fostering these skills, educators can empower students to become confident, critical thinkers who can apply their mathematical knowledge to solve real-world problems.

Understand the Problem

Breaking down the problem and identifying the key components.

To improve problem-solving math skills, it’s essential to first understand the problem at hand. Here are some tips to help break down the problem and identify its key components:

1. Read the problem carefully:  Take your time to read it attentively and ensure you understand what it asks. Pay attention to keywords or phrases that indicate what mathematical operation or concept to use.

2. Identify the known and unknown variables:  Determine what information is already given in the problem (known variables) and what you need to find (unknown variables). This step will help you analyze the problem more effectively.

3. Define the problem in your own words:  Restate the problem using your own words to ensure you clearly understand what needs to be solved. It can help you focus on the main objective and eliminate any distractions.

4. Break the problem into smaller parts:  Complex math problems can sometimes be overwhelming. Breaking them down into smaller, manageable parts can make them more approachable. Identify any sub-problems or intermediate steps that must be solved before reaching the final solution.

Reading and interpreting math word problems effectively

Many math problems are presented as word problems requiring reading and interpreting skills. Here are some strategies to help you effectively understand and solve math word problems:

1. Highlight key information:  As you read the word problem, underline or highlight any important details, such as numbers, units of measurement, or specific keywords related to mathematical operations.

2. Visualize the problem:  Create visual representations, such as diagrams or graphs, to help you understand the problem better. Visualizing the problem can make determining what steps to take and how to approach the solution easier.

3. Translate words into equations:  Convert the information in the word problem into mathematical equations or expressions. This translation step helps you transform the problem into a solvable math equation.

4. Solve step by step:  Break down the problem into smaller steps and solve each step individually. This approach helps you avoid confusion and progress toward the correct solution.

Improving problem-solving skills in math requires practice and patience. By understanding the problem thoroughly, breaking it into manageable parts, and effectively interpreting word problems, you can confidently enhance your ability to solve math problems.

Use Visual Representations

Using diagrams, charts, and graphs to visualize the problem.

One effective way to improve problem-solving skills in math is to utilize visual representations. Visual representations , such as diagrams, charts, and graphs, can help make complex problems more tangible and easily understood. Here are some ways to use visual representations in problem-solving:

1. Draw Diagrams:  When faced with a word problem or a complex mathematical concept, drawing a diagram can help break down the problem into more manageable parts. For example, suppose you are dealing with a geometry problem. In that case, sketching the shapes involved can provide valuable insights and help you visualize the problem better.

2. Create Charts or Tables:  For problems that involve data or quantitative information, creating charts or tables can help organize the data and identify patterns or trends. It can be particularly useful in analyzing data from surveys, experiments, or real-life scenarios.

3. Graphical Representations:  Graphs can be powerful tools in problem-solving, especially when dealing with functions, equations, or mathematical relationships. Graphically representing data or equations makes it easier to identify key features that may be hard to spot from a numerical representation alone, such as intercepts or trends.

Benefits of visual representation in problem-solving

Using visual representations in problem-solving offers several benefits:

1. Enhances Comprehension:  Visual representations provide a visual context for abstract mathematical concepts, making them easier to understand and grasp.

2. Encourages Critical Thinking:  Visual representations require active engagement and critical thinking skills. Students can enhance their problem-solving and critical thinking abilities by analyzing and interpreting visual data.

3. Promotes Pattern Recognition: Visual representations simplify identifying patterns, trends, and relationships within data or mathematical concepts. It can lead to more efficient problem-solving and a deeper understanding of mathematical principles.

4. Facilitates Communication:  Visual representations can be shared and discussed, helping students communicate their thoughts and ideas effectively. It can be particularly useful in collaborative problem-solving environments.

Incorporating visual representations into math problem-solving can significantly enhance understanding, critical thinking, pattern recognition, and communication skills. Students can approach math problems with a fresh perspective and improve their problem-solving abilities using visual tools.

Work Backwards

Understanding the concept of working backward in math problem-solving.

Working backward is a problem-solving strategy that starts with the solution and returns to the given problem. This approach can be particularly useful in math, as it helps students break down complex problems into smaller, more manageable steps. Here’s how to apply the concept of working backward in math problem-solving:

1. Identify the desired outcome : Start by clearly defining the goal or solution you are trying to reach. It could be finding the value of an unknown variable, determining a specific measurement, or solving for a particular quantity.

2. Visualize the result : Imagine the final step or solution. It will help you create a mental image of the steps needed to reach that outcome.

3. Trace the steps backward : Break down the problem into smaller steps, working backward from the desired outcome. Think about what needs to happen immediately before reaching the final solution and continue tracing the steps back to the beginning of the problem.

4. Check your work : Once you have worked backward to the beginning of the problem, double-check your calculations and steps to ensure accuracy.

Real-life examples and applications of working backward

Working backward is a valuable problem-solving technique in math and has real-life applications. Here are a few examples:

1. Financial planning : When creating a budget, you can work backward by determining your desired savings or spending amount and then calculating how much income or expenses are needed to reach that goal.

2. Project management : When planning a project, you can work backward by setting a fixed deadline and then determining the necessary steps and timelines to complete the project on time.

3. Game strategy : In games like chess or poker, working backward can help you anticipate your opponent’s moves and plan your strategy accordingly.

4. Recipe adjustments : When modifying a recipe, you can work backward by envisioning the final taste or texture you want to achieve and adjusting the ingredients or cooking methods accordingly.

By practicing working backward in math and applying it to real-life situations, you can enhance your problem-solving abilities and find creative solutions to various challenges.

Try Different Strategies

When solving math problems, it’s essential to have a repertoire of problem-solving strategies. You can improve your problem-solving skills and tackle various mathematical challenges by trying different approaches. Here are some strategies to consider:

Exploring Various Problem-Solving Strategies

1. Guess and Check:  This strategy involves making an educated guess and checking if it leads to the correct solution. It can be useful when dealing with trial-and-error problems.

2. Drawing a Diagram:  Visually representing the problem through diagrams or graphs can help you understand and solve it more effectively. This strategy is particularly useful in geometry and algebraic reasoning.

3. Using Logic:  Using logical reasoning is useful for breaking down complicated problems into smaller, more manageable components. This strategy is especially useful in mathematical proofs and logical puzzles.

4. Working Backwards:  Start with the desired outcome and return to the given information. When dealing with equations or word problems, this approach can assist.

5. Using Patterns:  Look for patterns and relationships within the problem to determine a solution. This approach can be used for different mathematical problems, such as sequences and numerical patterns.

When and How to Apply Different Strategies in Math Problem-Solving

Knowing when and how to apply different problem-solving strategies is crucial for success in math. Here are some tips:

• Understand the problem: Read the problem carefully and identify the key information and requirements.
• Select an appropriate strategy: Choose the most appropriate problem-solving strategy for the problem.
• Apply the chosen strategy: Implement the selected strategy, following the necessary steps.
• Check your solution: Verify your answer by double-checking the calculations or applying alternative methods.
• Reflect on the process: After solving the problem, take a moment to reflect and evaluate your problem-solving approach. Identify areas for improvement and consider alternative strategies that could have been used.

By exploring different problem-solving strategies and applying them to various math problems, you can enhance your problem-solving skills and develop a versatile toolkit for tackling mathematical challenges. Practice and persistence are key to honing your problem-solving abilities in math.

Key takeaways and tips for improving problem-solving skills in math

In conclusion, developing strong problem-solving skills in math is crucial for success in this subject. Here are some key takeaways and tips to help you improve your problem-solving abilities:

• Practice regularly:  The more you practice solving math problems, the better you will become at identifying patterns, applying strategies, and finding solutions.
• Break down the problem:  When faced with a complex math problem, break it into smaller, more manageable parts. It will make it easier to understand and solve.
• Understand the problem:  Before diving into a solution, fully understand the problem. Identify what information is given and what you are asked to find.
• Draw diagrams or visualize:  Use visual aids, such as diagrams or sketches, to help you better understand the problem and visualize the solution.
• Use logical reasoning:  Apply logical reasoning skills to analyze the problem and determine the most appropriate approach or strategy.
• Try different strategies:  If one approach doesn’t work, don’t be afraid to try different strategies or methods. There are often multiple ways to solve a math problem.
• Seek help and collaborate:  Don’t hesitate to seek help from your teacher, classmates, or online resources. Collaborating with others can provide different perspectives and insights.
• Learn from mistakes:  Mistakes are a valuable learning opportunity. Analyze your mistakes, understand where you went wrong, and learn from them to avoid making the same errors in the future.
• Grade 6 Lessons
• Grade 7 Lessons
• Grade 8 Lessons
• Kindergarten
• Math Lessons Online
• Math Tutorial
• Multiplication
• Subtraction
• #basic mathematic
• #Basic Mathematical Operation
• #best math online math tutor
• #Best Math OnlineTutor
• #dividing fractions
• #effective teaching
• #grade 8 math lessons
• #linear equation
• #Math Online Blog
• #mathematical rule
• #mutiplying fractions
• #odd and even numbers
• #Online Math Tutor
• #online teaching
• #order of math operations
• #pemdas rule
• #Point-Slope Form
• #Precalculus
• #Slope-Intercept Form
• #Tutoring Kids

Thank you for signing up!

GET IN TOUCH WITH US

• 1st Grade Math
• 2nd Grade Math
• 3rd Grade Math
• 4th Grade Math
• 5th Grade Math
• 6th Grade Math
• 7th Grade Math
• 8th Grade Math
• Knowledge Base
• Math for kids

10 Strategies for Problem Solving in Math

Created on May 19, 2022

Updated on January 6, 2024

When faced with problem-solving, children often get stuck. Word puzzles and math questions with an unknown variable, like x, usually confuse them. Therefore, this article discusses math strategies and how your students may use them since instructors often have to lead students through this problem-solving maze.

What Are Problem Solving Strategies in Math?

If you want to fix a problem, you need a solid plan. Math strategies for problem solving are ways of tackling math in a way that guarantees better outcomes. These strategies simplify math for kids so that less time is spent figuring out the problem. Both those new to mathematics and those more knowledgeable about the subject may benefit from these methods.

There are several methods to apply problem-solving procedures in math, and each strategy is different. While none of these methods failsafe, they may help your student become a better problem solver, particularly when paired with practice and examples. The more math problems kids tackle, the more math problem solving skills they acquire, and practice is the key.

Strategies for Problem-solving in Math

Even if a student is not a math wiz, a suitable solution to mathematical problems in math may help them discover answers. There is no one best method for helping students solve arithmetic problems, but the following ten approaches have shown to be very effective.

Understand the Problem

Understanding the nature of math problems is a prerequisite to solving them. They need to specify what kind of issue it is ( fraction problem , word problem, quadratic equation, etc.). Searching for keywords in the math problem, revisiting similar questions, or consulting the internet are all great ways to strengthen their grasp of the material. This step keeps the pupil on track.

1:1 Math Lessons

Want to raise a genius? Start learning Math with Brighterly

Guess and check.

One of the time-intensive strategies for resolving mathematical problems is the guess and check method. In this approach, students keep guessing until they get the answer right.

After assuming how to solve a math issue, students should reintroduce that assumption to check for correctness. While the approach may appear cumbersome, it is typically successful in revealing patterns in a child’s thought process.

Work It Out

Encourage pupils to record their thinking process as they go through a math problem. Since this technique requires an initial comprehension of the topic, it serves as a self-monitoring method for mathematics students. If they immediately start solving the problem, they risk making mistakes.

Students may keep track of their ideas and fix their math problems as they go along using this method. A youngster may still need you to explain their methods of solving the arithmetic questions on the extra page. This confirmation stage etches the steps they took to solve the problem in their minds.

Work Backwards

In mathematics, a fresh perspective is sometimes the key to a successful solution. Young people need to know that the ability to recreate math problems is valuable in many professional fields, including project management and engineering.

Students may better prepare for difficulties in real-world circumstances by using the “Work Backwards” technique. The end product may be used as a start-off point to identify the underlying issue.

In most cases, a visual representation of a math problem may help youngsters understand it better. Some of the most helpful math tactics for kids include having them play out the issue and picture how to solve it.

One way to visualize a workout is to use a blank piece of paper to draw a picture or make tally marks. Students might also use a marker and a whiteboard to draw as they demonstrate the technique before writing it down.

Find a Pattern

Kids who use pattern recognition techniques can better grasp math concepts and retain formulae. The most remarkable technique for problem solving in mathematics is to help students see patterns in math problems by instructing them how to extract and list relevant details. This method may be used by students when learning shapes and other topics that need repetition.

Students may use this strategy to spot patterns and fill in the blanks. Over time, this strategy will help kids answer math problems quickly.

When faced with a math word problem, it might be helpful to ask, “What are some possible solutions to this issue?” It encourages you to give the problem more thought, develop creative solutions, and prevent you from being stuck in a rut. So, tell the pupils to think about the math problems and not just go with the first solution that comes to mind.

Draw a Picture or Diagram

Drawing a picture of a math problem can help kids understand how to solve it, just like picturing it can help them see it. Shapes or numbers could be used to show the forms to keep things easy. Kids might learn how to use dots or letters to show the parts of a pattern or graph if you teach them.

Charts and graphs can be useful even when math isn’t involved. Kids can draw pictures of the ideas they read about to help them remember them after they’ve learned them. The plan for how to solve the mathematical problem will help kids understand what the problem is and how to solve it.

Trial and Error Method

The trial and error method may be one of the most common problem solving strategies for kids to figure out how to solve problems. But how well this strategy is used will determine how well it works. Students have a hard time figuring out math questions if they don’t have clear formulas or instructions.

They have a better chance of getting the correct answer, though, if they first make a list of possible answers based on rules they already know and then try each one. Don’t be too quick to tell kids they shouldn’t learn by making mistakes.

Review Answers with Peers

It’s fun to work on your math skills with friends by reviewing the answers to math questions together. If different students have different ideas about how to solve the same problem, get them to share their thoughts with the class.

During class time, kids’ ways of working might be compared. Then, students can make their points stronger by fixing these problems.

Check out the Printable Math Worksheets for Your Kids!

There are different ways to solve problems that can affect how fast and well students do on math tests. That’s why they need to learn the best ways to do things. If students follow the steps in this piece, they will have better experiences with solving math questions.

Jessica is a a seasoned math tutor with over a decade of experience in the field. With a BSc and Master’s degree in Mathematics, she enjoys nurturing math geniuses, regardless of their age, grade, and skills. Apart from tutoring, Jessica blogs at Brighterly. She also has experience in child psychology, homeschooling and curriculum consultation for schools and EdTech websites.

As adults, we take numbers for granted, but preschoolers and kindergartners have no idea what these symbols mean. Yet, we often demand instant understanding and flawless performance when we start teaching numbers to our children. If you don’t have a clue about how to teach numbers for kids, browse no more. You will get four […]

May 19, 2022

Teaching children is a complex process because they require more attention than an adult person. You may need to employ different teaching strategies when teaching kids. But what are teaching strategies? Teaching strategies are the methods to ensure your kids or students learn efficiently. But not all strategies yield similarly, and if the one you […]

Book 1 to 1 Math Lesson

Kid’s grade

After-School Math Program Boost Your Child's Math Abilities! Ideal for 1st-12th Graders, Perfectly Synced with School Curriculum!

After-School Math Program

Related posts.

Types of Assessment that Allow to Determine the Level of Students’ Knowledge

Assessment is a routine evaluation of students’ understanding of concepts and their needs for achieving success in studying. Assessment is critical in teaching because it amplifies students’ strengths and weaknesses. This article will show you how effective assessment methods help students reach the highest academic standards. What Is the Purpose of Assessment? Teachers can promote […]

What is Mental Math and Why is It Important for Children?

Teachers usually use strategies like games and worksheets to ensure that kids find math an easy subject. Mental math is one of the strategies that can help your kids develop their foundational math skills. But before delving into the benefits of mental math for your child, let’s answer the question “what does mental math mean?” […]

Apr 05, 2022

The Benefits of One on One Tutoring for Kids’ Development

Schools have changed significantly over the years, both in terms of structure and the process of learning. After-school support to boost kids’ performance has become more necessary. Knowing that extra help will set their kids on the path to mastery of school subjects, parents enlist professional tutors. So, if you are looking to do the […]

May 25, 2022

We use cookies to help give you the best service possible. If you continue to use the website we will understand that you consent to the Terms and Conditions. These cookies are safe and secure. We will not share your history logs with third parties. Learn More

Or search by topic

Number and algebra

• The Number System and Place Value
• Calculations and Numerical Methods
• Fractions, Decimals, Percentages, Ratio and Proportion
• Properties of Numbers
• Patterns, Sequences and Structure
• Algebraic expressions, equations and formulae
• Coordinates, Functions and Graphs

Geometry and measure

• Angles, Polygons, and Geometrical Proof
• 3D Geometry, Shape and Space
• Measuring and calculating with units
• Transformations and constructions
• Pythagoras and Trigonometry
• Vectors and Matrices

Probability and statistics

• Handling, Processing and Representing Data
• Probability

Working mathematically

• Thinking mathematically
• Mathematical mindsets
• Cross-curricular contexts
• Physical and digital manipulatives

For younger learners

• Early Years Foundation Stage

Advanced mathematics

• Decision Mathematics and Combinatorics
• Advanced Probability and Statistics

A Guide to Problem Solving

When confronted with a problem, in which the solution is not clear, you need to be a skilled problem-solver to know how to proceed. When you look at STEP problems for the first time, it may seem like this problem-solving skill is out of your reach, but like any skill, you can improve your problem-solving with practice. How do I become a better problem-solver? First and foremost, the best way to become better at problem-solving is to try solving lots of problems! If you are preparing for STEP, it makes sense that some of these problems should be STEP questions, but to start off with it's worth spending time looking at problems from other sources. This collection of NRICH problems  is designed for younger students, but it's very worthwhile having a go at a few to practise the problem-solving technique in a context where the mathematics should be straightforward to you. Then as you become a more confident problem-solver you can try more past STEP questions. One student who worked with NRICH said: "From personal experience, I was disastrous at STEP to start with. Yet as I persisted with it for a long time it eventually started to click - 'it' referring to being able to solve problems much more easily. This happens because your brain starts to recognise that problems fall into various categories and you subconsciously remember successes and pitfalls of previous 'similar' problems." A Problem-solving Heuristic for STEP Below you will find some questions you can ask yourself while you are solving a problem. The questions are divided into four phases, based loosely on those found in George Pólya's 1945 book "How to Solve It". Understanding the problem

• What area of mathematics is this?
• What exactly am I being asked to do?
• What do I know?
• What do I need to find out?
• What am I uncertain about?
• Can I put the problem into my own words?

Devising a plan

• Work out the first few steps before leaping in!
• Have I seen something like it before?
• Is there a diagram I could draw to help?
• Is there another way of representing?
• Would it be useful to try some suitable numbers first?
• Is there some notation that will help?

Carrying out the plan STUCK!

• Try special cases or a simpler problem
• Work backwards
• Guess and check
• Be systematic
• Work towards subgoals
• Imagine your way through the problem
• Has the plan failed? Know when it's time to abandon the plan and move on.

Looking back

• Have I answered the question?
• Sanity check for sense and consistency
• Check the problem has been fully solved
• Read through the solution and check the flow of the logic.

Throughout the problem solving process it's important to keep an eye on how you're feeling and making sure you're in control:

• Am I getting stressed?
• Is my plan working?
• Am I spending too long on this?
• Could I move on to something else and come back to this later?
• Am I focussing on the problem?
• Is my work becoming chaotic, do I need to slow down, go back and tidy up?
• Do I need to STOP, PEN DOWN, THINK?

Finally, don't forget that STEP questions are designed to take at least 30-45 minutes to solve, and to start with they will take you longer than that. As a last resort, read the solution, but not until you have spent a long time just thinking about the problem, making notes, trying things out and looking at resources that can help you. If you do end up reading the solution, then come back to the same problem a few days or weeks later to have another go at it.

5 Ways to Build Math Problem Solving Skills (based on brain research)

Whether talking about state tests or meeting with your team to plan the next math unit, the conversation inevitably turns to word problems. But knowing how to build math problem-solving skills without resorting to pages of boring story problem practice can be hard.

These days word problems aren’t the basic one-step wonders that many of us dealt with as students. Instead, multi-step story problems that require students to apply multiple concepts and skills are incorporated into instruction and state assessments.

Understanding brain research can help simply the process of teaching this challenging format of math problem-solving to students, including those who struggle.

What research says about building master problem solvers in math

Have you seen how many math skills we must teach these days? No teacher has enough time to build critical math skills AND effectively teach problem-solving…or do they?

Research would argue we are going about these tasks all wrong. They say there are many reasons students struggle with math word problems , but one big one is that we aren’t doing what’s best for the brain. Instead, here’s what the brain research says about the must-have elements for building step-by-step math problem-solving mastery.

Finding #1: Becoming a master problem solver requires repetition.

Duh, right? Any good teacher knows this…but what’s the best recipe for repetition if you want students to master math word problems? How much practice? How often?

Let’s start with the concept of mastery.

How do you develop math problem solving skills?

In the 1990’s, Anders Ericsson studied experts to explore what made some people excel. Findings showed a positive correlation between the amount of deliberate practice (activities that require a high level of concentration and aren’t necessarily inherently fun) and skill level.

In other words, the more practice someone gets, the more they improve. This became the basis of Malcolm Gladwell’s 10,000-hour rule, which stated that it takes 10,000 hours to make you an expert in a field.

But what should that practice look like for students who struggle with word problems? Is it better to have a deep dive into story problems, or do short bursts of practice do more for long-term understanding?

Designing Better Word Problem Activities: Building Step-by-step Math Problem-Solving Practice

We can look at Ebbinghaus’ work on memory & retention to answer that.  He found spacing practice over time decreased the number of exposures needed. In other words, small amounts of practice over several days, weeks, or even months actually means you need LESS practice than if you try to cram it all in at once.

For over 80 years, this finding has stood the test of time. While research has shown that students who engage in mass practice (lots of practice all at once) might do better on an assessment that takes place tomorrow, students who engage in repeated practice over a period of time retain more skills long-term (Bloom & Shuell, 1981; Rea & Modigliani, 1985).

And how long does the research say you should spend reviewing?

How long should problem-solving practice really be?

Shorter is better. As discussed earlier, peak attention required for deliberate practice can only be maintained for so long. And the majority of research supports 8-10 minutes as the ideal lesson length (Robertson, 2010).

This means practice needs to be focused so that during those minutes of discussion, you can dive deep – breaking down the word problem and discussing methods to solve it.

Teacher Tip: Applying this finding to your classroom

Less is actually more as long as you plan to practice regularly. While students who struggle with word problems may need a great deal of practice to master word problems, ideally, this practice should be provided in short, regular intervals with no more than 8-10 minutes spent in whole group discussion.

Here are a few simple steps to apply these findings to your math classroom:

• Find 8-12 minutes in your daily schedule to focus on problem-solving – consider this time sacred & only for problem-solving.
• Select only 1-2 word problems per day. Target step-by-step math problem-solving to build math problem-solving skills through a less-is-more approach using Problem of the Day .

Finding #2: Students who are challenged & supported have better outcomes.

Productive struggle, as it is called in the research, focuses on the effortful practice that builds long-term understanding.

Important to this process are opportunities for choice, collaboration, and the use of materials or topics of interest (which will be discussed later).

This productive struggle also helps students build flexible thinking so that they can apply previously learned skills to new or unfamiliar tasks (Bransford, Brown, & Cocking, 2000).

“Meaningful learning tasks need to challenge ever student in some way. It is crucial that no student be able to coast to success time after time; this experience can create the belief that you are smart only if you can succeed without effort.” -Carol Dweck

It is also critical to provide support and feedback during the challenging task (Cimpian, Arce, Markman, & Dweck, 2007). This prevents frustration and fear of failure when the goal seems out of reach or when a particularly challenging task arises.

Simple ways to build productive struggle into your math classroom

Giving students who struggle with word problems a chance to struggle with challenging word problems is critical to building confidence and skills. However, this challenge must be reasonable, or the learner’s self-esteem will falter, and students need support and regular feedback to achieve their potential.

Here are a few simple things to try:

• Select problems that are just at the edge of students’ Zone of Proximal Development.
• Scaffold or model with more challenging problems to support risk-taking.
• Give regular feedback & support – go over the work and discuss daily.

Finding #3: Novelty & variation are keys to engagement.

When it comes to standardized testing (and life in general), problems that arise aren’t labeled with the skills and strategies required to solve them.

This makes it important to provide mixed practice opportunities so students are focused on asking themselves questions about what the problem is asking and what they are trying to find.

This type of variation not only supports a deeper level of engagement, it also supports the metacognitive strategies needed to analyze and develop a strategy to solve (Rohrer & Taylor, 2014).

The benefits of novelty in learning

A 2013 study also supports the importance of novelty in supporting reinforcement learning (aka review). The findings suggested that when task variation was provided for an already familiar skill, it offered the following benefits:

• reduced errors due to lack of focus
• helped learners maintain attention to task
• motivated and engaged student

Using variety to build connections & deepen understanding

In addition, by providing variations in practice, we can also help learners understand the skills and strategies they are using on a deeper level.  

When students who struggle with word problems are forced to apply their toolbox of strategies to novel problem formats, they begin to analyze and observe patterns in how problems are structured and the meaning they bring.

This requires much more engagement than being handed a sheet full of multiplication story problems, where students can pull the numbers and compute with little focus on understanding.

Designing word problems that incorporate variety & novelty

Don’t be afraid to shake things up!

Giving students practice opportunities with different skills or problem formats mixed in is a great way to boost engagement and develop meta-cognitive skills.

Here are a few tips for trying it out in the classroom:

• Change it up! Word problem practice doesn’t have to match the day’s math lesson.
• Give opportunities to practice the same skill or strategy in via different formats.
• Adjust the wording and/or topic in word problems to help students generalize skills.

Finding #4: Interest and emotion increase retention and skill development.

Attention and emotion are huge for learning. We’ve all seen it in our classroom.

Those magical lessons that hook learners are the ones that stick with them for years to come, but what does the research say?

The Science Behind Emotion & Learning

Neuroscientists have shown that emotions create connections among different sections of the brain (Immordino-Yang, 2016) . This supports long-term retrieval of the skills taught and a deeper connection to the learning.

This means if you can connect problem-solving with a scenario or a feeling, your students will be more likely to internalize the skills being practiced. Whether this is by “wowing” them with a little-known fact or solving real-world problems, the emotional trigger can be huge for learning.

What about incorporating student interests?

As for student interests, a long line of research supports the benefits of using these to increase educational outcomes and student motivation, including for students who struggle with word problems (Chen, 2001; Chen & Ennis, 2004; Solomon, 1996).

Connecting classwork with student interests has increased students’ intentions to participate in future learning endeavors (Chen, 2001).

And interests don’t just mean that love of Pokemon!

It means allowing social butterflies to work collaboratively. Providing students with opportunities to manipulate real objects or create models. Allowing kids to be authentic while digging in and developing the skills they need to master their learning objectives.

What this looks like in a math class

Evoke emotion and use student interests to engage the brain in deep, long-lasting learning whenever possible.

This will help with today’s learning and promote long-term engagement, even when later practice might not be as interesting for students who struggle with word problems.

Here’s how to start applying this research today:

• Find word problems that match student interests.
• Connect real-life situations and emotions to story problem practice.
• Consider a weekly theme to connect practice throughout the week.

Finding #5: Student autonomy builds confidence & independence.

Autonomy is a student’s ability to be in control of their learning. In other words, it is their ability to take ownership over the learning process and how they demonstrate mastery.

Why students need to control their learning

Research shows that providing students a sense of control and supporting their choices is way to help engage learners and build independent thinking. It also increased intrinsic motivation (Reeve, Nix, & Hamm, 2003).

However, this doesn’t mean we just let kids learn independently. Clearly, some things require repeated guidance and modeling. Finding small ways that students can take control of the learning process is much better in these instances.

We know that giving at least partial autonomy has been linked to numerous positive student learning outcomes (Wielenga-Meijer, Taris, Widboldus, & Kompier, 2011).

But how can we foster this independence and autonomy, especially with those students who struggle to self-regulate behavior?

Fostering independence in students who struggle to stay on task

Well, the research says several conditions support building toward independence.

The first (and often neglected) is to explain unappealing choices and why they are one of the options.

When it comes to word problems, this might include explaining the rationale behind one of the strategies that appears to be a lot more work than the others.

It is also important to acknowledge students’ negative feelings about a task or their ability to complete it. While we want them to be able to build independence, we don’t want them to drown in overwhelm.

By providing emotional support, we can help determine whether a student is stuck with the learning or with the emotions from the cognitive challenge.

Finally, giving choices is recommended. Identifying choices you and your students who struggle with word problems can live with is an important step.

Whether this is working in partners, trying an alternative method, or skipping a problem and coming back, students need to feel like they have some ownership over the challenge they are working through.

By building in opportunities for autonomy, and choice, teachers help students build a sense of self-efficacy and confidence in their ability to be successful learners across various contexts (McCombs, 2002,2006).

We know this leads to numerous positive outcomes and has even been linked to drop-out prevention (Christenson & Thurlow, 2004).

Fostering autonomy in your classroom

You’re not going to be able to hold their hands forever.

Giving opportunities to work through challenges independently and to feel ownership for their choices will help build both confidence and skills.

Here’s how to get started letting go:

• Give students time to tackle the problem independently (or in partners).
• Don’t get hyper-focused on a single method to solve – give opportunities to share & learn together.
• Provide appropriate support (where needed) to build autonomy for all learners – like reading the problem orally.

Finding #6: Students need to be taught how to fail & recover from it.

Despite Ericsson’s findings discussed early on in this post, talent does matter, and it is important to teach students to recover from failure because those are the moments when they learn the most.

A 2014 study by Brooke Macnamara analyzed 88 studies to determine how talent factored into deliberate practice.

Her findings show what we (as teachers) already know, students may require different amounts of practice to reach the same skill level…but how do we keep those struggling students from keeping up?

Growth mindset research gives us insight into ways to support students who struggle with word problems, encourage all students in math problem-solving, and harness the power of failure through “yet.”

You might not be able to do something yet, but if you keep trying, you will. This opens the door for multiple practice opportunities where students learn from each other.

And what about the advanced students?

Many of these students have not experienced failure, but they may have met their match when it comes to complex word problems.

To support these students, who may be experiencing their first true challenge, we need to have high standards and provide constructive, supportive feedback on how to grow.

Then we need to give them space to try again.

There is great power in allowing students to revise and try again, but our grading system often discourages being comfortable with failure.

Building the confidence to fail in your classroom

Many students feel the pressure always to have the right answer. Allowing students to fail safely means you can help them learn from these failures so they don’t make the same mistake twice.

Here’s how you can safely foster growth and build math problem solving skills through failure in your classroom:

• Build in time to analyze errors & reflect.
• Reward effort & growth as much as, if not more than, accuracy.
• ​At least initially, skip the grading so students aren’t afraid to be wrong.

Getting started with brain-based problem solving

The brain research is clear.

Spending 45 minutes focused on a sheet of word problems following the same format isn’t the answer.

By implementing this research, you can save yourself time and the frustration from a disengaged class.

Based on this research, I’ve created Daily Problem Solving bundles to save you time and build math problem-solving skills. You can get each month separately or buy the full-year bundle at a major discount.

Currently, I offer these bundles for several grade levels, including:

Try Daily Problem Solving with your Learners

Of course, you do! Start working to build step-by-step math problem-solving skills today by clicking the button below to sign up for a free set of Daily Problem Solving.

Leave a comment Cancel reply

You must be logged in to post a comment.

Teaching Problem Solving in Math

• Freebies , Math , Planning

Every year my students can be fantastic at math…until they start to see math with words. For some reason, once math gets translated into reading, even my best readers start to panic. There is just something about word problems, or problem-solving, that causes children to think they don’t know how to complete them.

Every year in math, I start off by teaching my students problem-solving skills and strategies. Every year they moan and groan that they know them. Every year – paragraph one above. It was a vicious cycle. I needed something new.

I put together a problem-solving unit that would focus a bit more on strategies and steps in hopes that that would create problem-solving stars.

The Problem Solving Strategies

First, I wanted to make sure my students all learned the different strategies to solve problems, such as guess-and-check, using visuals (draw a picture, act it out, and modeling it), working backward, and organizational methods (tables, charts, and lists). In the past, I had used worksheet pages that would introduce one and provide the students with plenty of problems practicing that one strategy. I did like that because students could focus more on practicing the strategy itself, but I also wanted students to know when to use it, too, so I made sure they had both to practice.

I provided students with plenty of practice of the strategies, such as in this guess-and-check game.

There’s also this visuals strategy wheel practice.

I also provided them with paper dolls and a variety of clothing to create an organized list to determine just how many outfits their “friend” would have.

Then, as I said above, we practiced in a variety of ways to make sure we knew exactly when to use them. I really wanted to make sure they had this down!

Anyway, after I knew they had down the various strategies and when to use them, then we went into the actual problem-solving steps.

The Problem Solving Steps

I wanted students to understand that when they see a story problem, it isn’t scary. Really, it’s just the equation written out in words in a real-life situation. Then, I provided them with the “keys to success.”

S tep 1 – Understand the Problem.   To help students understand the problem, I provided them with sample problems, and together we did five important things:

• read the problem carefully
• restated the problem in our own words
• crossed out unimportant information
• circled any important information
• stated the goal or question to be solved

We did this over and over with example problems.

Once I felt the students had it down, we practiced it in a game of problem-solving relay. Students raced one another to see how quickly they could get down to the nitty-gritty of the word problems. We weren’t solving the problems – yet.

Then, we were on to Step 2 – Make a Plan . We talked about how this was where we were going to choose which strategy we were going to use. We also discussed how this was where we were going to figure out what operation to use. I taught the students Sheila Melton’s operation concept map.

We talked about how if you know the total and know if it is equal or not, that will determine what operation you are doing. So, we took an example problem, such as:

Sheldon wants to make a cupcake for each of his 28 classmates. He can make 7 cupcakes with one box of cupcake mix. How many boxes will he need to buy?

We started off by asking ourselves, “Do we know the total?” We know there are a total of 28 classmates. So, yes, we are separating. Then, we ask, “Is it equal?” Yes, he wants to make a cupcake for EACH of his classmates. So, we are dividing: 28 divided by 7 = 4. He will need to buy 4 boxes. (I actually went ahead and solved it here – which is the next step, too.)

Step 3 – Solving the problem . We talked about how solving the problem involves the following:

• taking our time
• working the problem out
• showing all our work
• estimating the answer
• using thinking strategies

We talked specifically about thinking strategies. Just like in reading, there are thinking strategies in math. I wanted students to be aware that sometimes when we are working on a problem, a particular strategy may not be working, and we may need to switch strategies. We also discussed that sometimes we may need to rethink the problem, to think of related content, or to even start over. We discussed these thinking strategies:

• switch strategies or try a different one
• rethink the problem
• think of related content
• decide if you need to make changes
• check your work
• but most important…don’t give up!

To make sure they were getting in practice utilizing these thinking strategies, I gave each group chart paper with a letter from a fellow “student” (not a real student), and they had to give advice on how to help them solve their problem using the thinking strategies above.

Finally, Step 4 – Check It.   This is the step that students often miss. I wanted to emphasize just how important it is! I went over it with them, discussing that when they check their problems, they should always look for these things:

• compare your answer to your estimate
• check for reasonableness
• check your calculations
• add the units
• restate the question in the answer
• explain how you solved the problem

Then, I gave students practice cards. I provided them with example cards of “students” who had completed their assignments already, and I wanted them to be the teacher. They needed to check the work and make sure it was completed correctly. If it wasn’t, then they needed to tell what they missed and correct it.

To demonstrate their understanding of the entire unit, we completed an adorable lap book (my first time ever putting together one or even creating one – I was surprised how well it turned out, actually). It was a great way to put everything we discussed in there.

Once we were all done, students were officially Problem Solving S.T.A.R.S. I just reminded students frequently of this acronym.

Stop – Don’t rush with any solution; just take your time and look everything over.

Think – Take your time to think about the problem and solution.

Act  – Act on a strategy and try it out.

Review – Look it over and see if you got all the parts.

Wow, you are a true trooper sticking it out in this lengthy post! To sum up the majority of what I have written here, I have some problem-solving bookmarks FREE to help you remember and to help your students!

You can grab these problem-solving bookmarks for FREE by clicking here .

You can do any of these ideas without having to purchase anything. However, if you are looking to save some time and energy, then they are all found in my Math Workshop Problem Solving Unit . The unit is for grade three, but it  may work for other grade levels. The practice problems are all for the early third-grade level.

• freebie , Math Workshop , Problem Solving

FIND IT NOW!

Check me out on tpt.

CHECK THESE OUT

Three Types of Rocks and Minerals with Rock Cycle Circle Book

Partitioning Shapes Equal Share Fractions Halves, Thirds, Fourths Math Puzzles

Want to save time?

COPYRIGHT © 2016-2024. The Owl Teacher | Privacy page | Disclosure Page | Shipping | Returns/Refunds

BOGO on EVERYTHING!

Need to Boost Your Math Skills? Try Gaming.

Just like math, gamification is rooted in problem-solving and perseverance.

If you are not a math person, consider boosting your skills for your career’s sake. The market for jobs requiring advanced math skills is expected to grow faster than other occupations through 2032, according to the U.S. Bureau of Labor Statistics . 

6 Games to Sharpen Your Math Skills

• Khan Academy  
• Buzzmath  

If you’re not a math person and would like to be, consider gaming to boost your skills. Educational games for adults integrate game mechanics into the teaching-learning process, with the intrinsic structure of the game promoting problem-solving skills .

A real plus Why Math Is Vital to Thrive in Your AI Career

How Games Help With Math

In essence, games are built around a core narrative or a mission where the gamer must conquer challenges or puzzles that require logical reasoning, strategy formation and decision making. These abilities are indispensable when learning math. 

Just like math, gamification is rooted in problem-solving and perseverance. Gamers confront a connected flow of challenges based on their real-time decisions rather than engaging in isolated problems.

Gamified environments present players with obstacles that require them to make quick assessments and judgments. Their decisions result in immediate feedback and consequences, but they play on whether they fail or succeed because the game doesn’t stop. The game presents the next stimulus that requires the next response, and as that stimulus-response loop repeats, learners build problem-solving skills and perseverance. 

A well-designed game is challenging and also encourages creative and strategic thinking challenges learners while encouraging them to think creatively and strategically, enabling them to apply, analyze, synthesize and evaluate various problems. 

Many educational games also encourage effective teamwork, bolstering adults’ collaborative problem-solving skills. This practice deepens their understanding of mathematical concepts by permitting them to explore different perspectives and solutions.

6 Games That Boost Math Skills

Educational gamification makes acquiring and mastering essential math skills an engaging and even addictive experience. Gamified math tools make complex concepts more accessible and learning math more enjoyable.  Here are six examples of educational gamification platforms designed for adults.

While not a traditional game, Photomath gamifies learning by offering instant problem-solving gratification. Users simply take a picture of a handwritten or printed math problem, and Photomath instantly provides a step-by-step solution. For working adults grappling with calculus, algebra or trigonometry, this app can demystify complex problems and reinforce learning through real-time solutions and explanations.

Brilliant  caters to a wide range of ages and skill levels, making it particularly useful for employed professionals seeking to sharpen their math skills. Brilliant uses interactive explorations and a problem-based approach to teach mathematical concepts, offering an array of courses including algebra, number theory and calculus. Users build knowledge through story-like narratives and active problem solving, which can be both challenging and rewarding.

Khan Academy

Khan Academy remains a standout resource for self-paced learning, with its extensive library of practice exercises, instructional videos, and a personalized learning dashboard. Adults can specifically benefit from its advanced math courses through gamification elements such as earning badges and points as they achieve goals, making the experience more interactive and motivating.

Buzzmath provides more than 7,000 activities that align with common mathematical skill standards. With a focus on guiding learners through a storyline and offering immediate detailed feedback, Buzzmath adds a game-like structure to math practice. The goal is to help learners build confidence and proficiency in key math areas, with content continually being updated to challenge and stimulate the user.

Mangahigh takes a unique approach to learning, blending anime-style narratives with rigorous math challenges that appeal to adult learners. Offering a wide range of games and activities that cover everything from basic algebra to advanced calculus , this platform boasts a competitive edge that can hook users as they can compete against peers in math duels, pushing them to improve their skills.

DragonBox Elements & Algebra

While typically associated with younger audiences, DragonBox offers advanced apps such as DragonBox Algebra 12+ and DragonBox Elements that are suitable for adult learners. These apps introduce mathematical concepts in a game environment, allowing learners to solve puzzles that indirectly teach algebra and geometry principles.

related reading Why You Need Critical Thinking Skills

More Tips for Boosting Your Math Skills

Games that boost math skills can be fun and perhaps frustrating as you develop skills. Here are two extra tips for boosting your math knowledge.

Revisit the Basics

If you feel your education didn’t give you a proper foundation in essential math skills, revisit the basics — addition, subtraction, multiplication and division. Math skills build off one another, so it is always best to start at the beginning. 

Ground yourself in a solid understanding of the basics before moving into more advanced skills like algebra or geometry . Even the higher relevant adult math skills center around understanding addition, subtraction, multiplication, division, fractions, negative numbers and basic algebra.

The path to acquiring essential math skills for an adult can be a long and laborious road. Attempting to learn everything all at once can be overwhelming, so approach the task with patience. For working professionals looking to improve their math skills, a little bit of learning each day is better than a five-hour study session once a month. 

Math doesn’t have to be mundane or intimidating. Adult learners have a range of innovative tools at their disposal to help them engage with and master essential math skills. Each of the platforms mentioned provides a unique take on gamification, using the inherent drives of competition, achievement and immediate feedback to redefine the math learning experience. Whether these tools are used inside or outside the classroom, they offer dynamic avenues for anyone looking to level up their math abilities.

Recent Expert Contributors Articles

How to Improve your Students’ Confidence with Problem Solving Skills in Math

• March 17, 2021

Hey guys!  I’m back with more information on how I work with my students on their problem-solving skills in my math classroom.  In case you missed it I shared my 5 easy steps to solve ANY word problem in my last blog post.   

So in case, you’re wondering?  Robin why are you repeating yourself?!  Well, if you haven’t been here before let me welcome you into my little space of all things random repeating math topics!  

In case you need a summary, most students struggle with word problems and do not even attempt to try to solve them! 

The exact phrases I have heard include:  “I don’t know how to do this!” Even when they just solved several problems on the exact same skill set.  

The best is when you see an IDK written on their paper!

How do we stop this craziness with students’ lack of confidence in their problem-solving skills?

How to Improve Mathematical Problem-Solving Skills

The first attempt at getting students to improve their mathematical problem-solving skills is to allow thinking to happen.  Too many times as teachers we don’t make time for word problems because they are tedious to teach and there is never a 1 size fits all approach to every problem.  

However, I have implemented a technique that can help students become successful problem solvers.  

Problem-Solving Techniques in Math

My method is based on the 4 steps basic method, but I break it down into 5 steps.  The main reason I am obsessed with my problem-solving method is 1 thing and 1 thing only:  Consistency!   

Students can stay consistent in problem-solving and be confident and successful at the same time.  

No more IDK’s on their papers! 

Problem-Solving Strategies in Mathematics

Like I mentioned in my last post we are going to help our students SOLVE each and every problem that they encounter with CONFIDENCE !

So what exactly does SOLVE stand for:

• S – State the objective 

 What is the question asking you?

• O – Outline your plan 

What problem-solving strategy are you going to use?  

• L – Look for Key Details – Information 

What keywords and or facts are needed or are extra. 

• V –  Verify and Solve

Verify your strategy and solve the problem

• E – Explain and check your solution

Write out a written explanation of your work and check that your solution makes sense.

Teaching Problem-Solving Strategies in Math

What exact strategies do you need to include in helping your students SOLVE any word problem that they encounter?  

A list of strategies that I use in my classroom include:

• Write an equation or use a formula
• Make it simpler
• Word backward
• Look for a pattern
• Make an organized list or table
• Make a model or Act it out
• Draw a picture or graph
• Logical thinking
• Divide and Conquer 
• Guess and Test

I’m going to be showing you how I do this process with my students in my first ever FREE Problem Solving Workshop! I’m super excited and I hope that you will join me! Don’t worry this workshop will be recorded and will live on forever and ever! 

This simple consistent problem-solving method will most definitely give your students the confidence to say I DO KNOW instead of I Don’t know!  

Need more ideas to motivate your students in the classroom?

Let me know if I’ll see you in my FREE workshop!

PS.  Need the SOLVE method for your bulletin board for your students’ math journals/notebooks?  Check out this bulletin board resource here:

PPS.   Need more problem-solving strategies in the classroom? I found this amazing article here !

• Latest Posts

Robin Cornecki

Latest posts by robin cornecki ( see all ).

• The #1 method for finding slope without using a formula! - April 25, 2023
• Here’s a Quick Way to Convert Percents to Fractions and Decimals. - July 21, 2022
• How to use the Four-Function Calculator for the Praxis Core Math Test.  - April 23, 2022

Hi, I'm Robin!

 I am a secondary math teacher with over 19 years of experience! If you’re a teacher looking for help with all the tips, tricks, and strategies for passing the praxis math core test, you’re in the right place!

I also create engaging secondary math resources for grades 7-12! 

Learn more about me and how I can help you here .

Let's Connect!

Get my top 7 strategies.

• PRO Courses Guides New Tech Help Pro Expert Videos About wikiHow Pro Upgrade Sign In
• EDIT Edit this Article
• EXPLORE Tech Help Pro About Us Random Article Quizzes Request a New Article Community Dashboard This Or That Game Popular Categories Arts and Entertainment Artwork Books Movies Computers and Electronics Computers Phone Skills Technology Hacks Health Men's Health Mental Health Women's Health Relationships Dating Love Relationship Issues Hobbies and Crafts Crafts Drawing Games Education & Communication Communication Skills Personal Development Studying Personal Care and Style Fashion Hair Care Personal Hygiene Youth Personal Care School Stuff Dating All Categories Arts and Entertainment Finance and Business Home and Garden Relationship Quizzes Cars & Other Vehicles Food and Entertaining Personal Care and Style Sports and Fitness Computers and Electronics Health Pets and Animals Travel Education & Communication Hobbies and Crafts Philosophy and Religion Work World Family Life Holidays and Traditions Relationships Youth
• Browse Articles
• Learn Something New
• Quizzes Hot
• This Or That Game
• Train Your Brain
• Explore More
• Support wikiHow
• About wikiHow
• Log in / Sign up
• Education and Communications
• Mathematics

How to Improve Mental Math Skills

Last Updated: May 9, 2024 Approved

This article was co-authored by Daron Cam . Daron Cam is an Academic Tutor and the Founder of Bay Area Tutors, Inc., a San Francisco Bay Area-based tutoring service that provides tutoring in mathematics, science, and overall academic confidence building. Daron has over eight years of teaching math in classrooms and over nine years of one-on-one tutoring experience. He teaches all levels of math including calculus, pre-algebra, algebra I, geometry, and SAT/ACT math prep. Daron holds a BA from the University of California, Berkeley and a math teaching credential from St. Mary's College. There are 8 references cited in this article, which can be found at the bottom of the page. wikiHow marks an article as reader-approved once it receives enough positive feedback. In this case, 83% of readers who voted found the article helpful, earning it our reader-approved status. This article has been viewed 311,567 times.

Eventually, you'll find yourself in a situation where you'll have to solve a math problem without a calculator. Trying to imagine a pen and paper in your head often doesn't help much. Fortunately there are faster and easier ways to do calculations in your head—and they often break down a problem in a way that makes more sense than what you learned in school. Whether you're a stressed-out student or a math wizard looking for even faster tricks, there's something for everyone to learn.

Break addition and subtraction problems into parts.

• 712 + 281 → "700 + 200," "10 + 80," and "2 + 1"
• 700 + 200 = 9 00, then 10 + 80 = 9 0, then 2 + 1 = 3
• 900 + 90 + 3 = 993 .
• Thinking in "hundreds" or "tens" instead of single digits will make it easier to keep track when digits sum to more than ten. For example, for 37 + 45, think "30 + 40 = 70" and "7 + 5 = 12". Then add 70 + 12 to get 82.

Change the problem to make round numbers.

• Addition : For 596 + 380 , realize that you can add 4 to 596 to round it to 600, then add 600 + 380 to get 980. Undo the rounding by subtracting 4 from 980 to get 976 .
• Subtraction : For 815 - 521 , break it up into 800 - 500, 10 - 20, and 5 - 1. To turn the awkward "10 - 20" into "20 - 20", add 10 to 815 to get 825. Now solve to get 304, then undo the rounding by subtracting 10 to get 294 .
• Multiplication : For 38 x 3 , you can add 2 to 38 to make the problem 40 x 3, which is 120. Since the 2 you added got multiplied by three, you need to undo the rounding by subtracting 2 x 3 = 6 at the end to get 120 - 6 = 114 .

Learn to add many numbers at once.

• For example, 7 + 4 + 9 + 13 + 6 + 51 can be reorganized to (7 + 13) + (9 + 51) + (6 + 4) = 20 + 60 + 10 = 90.

Multiply from left to right.

• For 453 x 4 , start with 400 x 4 = 1600, then 50 x 4 = 200, then 3 x 4 = 12. Add them all together to get 1812 .
• If both numbers have more than one digit, you can break it into parts. Each digit has to multiply with each other digit, so it can be tough to keep track of it all. 34 x 12 = (34 x 10) + (34 x 2) , which you can break down further into (30 x 10) + (4 x 10) + (30 x 2) + (4 x 2) = 300 + 40 + 60 + 8 = 408 .

Try a fast multiplication trick best for numbers 11 through 19.

• Let's look at numbers close to 10, like 13 x 15 . Subtract 10 from the second number, then add your answer to the first: 15 - 10 = 5, and 13 + 5 = 18.
• Multiply your answer by ten: 18 x 10 = 180.
• Next, subtract ten from both sides and multiply the results: 3 x 5 = 15.
• Add your two answers together to get the final answer: 180 + 15 = 195 .
• Careful with smaller numbers! For 13 x 8, you start with "8 - 10 = -2", then "13 + -2 = 11". If it's hard to work with negative numbers in your head, try a different method for problems like this.
• For larger numbers, it will be easier to use a "base number" like 20 or 30 instead of 10. If you try this, make sure you use that number everywhere that 10 is used above. [3] X Research source For example, for 21 x 24, you start by adding 21 + 4 to get 25. Now multiply 25 by 20 (instead of ten) to get 500, and add 1 x 4 = 4 to get 504.

Simplify problems with numbers ending in zero.

• Addition : If all numbers have zeroes at the end, you can ignore the zeroes they have in common and restore them at the end. 85 0 + 12 0 → 85 + 12 = 97, then restore the shared zero: 97 0 .
• Subtraction works the same way: 10 00 - 7 00 → 10 - 7 = 3, then restore the two shared zeroes to get 3 00 . Notice that you can only remove the two zeroes the numbers have in common, and must keep the third zero in 1000.
• Multiplication : ignore all the zeroes, then restore each one individually. 3 000 x 5 0 → 3 x 5 = 15, then restore all four zeroes to get 15 0 , 00 0 .
• Division : you can remove all shared zeroes and the answer will be the same. 60, 000 ÷ 12, 000 = 60 ÷ 12 = 5 . Don't add any zeroes back on.

Easily multiply by 4, 5, 8, or 16.

• To multiply by 5, instead multiply by 10, then divide by 2.
• To multiply by 4, instead double the number, then double it again.
• For 8, 16, 32, or even higher powers of two, just keep doubling. For example, 13 x 8 = 13 x 2 x 2 x 2, so double 13 three times: 13 → 26 → 52 → 104 .

Memorize the 11s trick.

• What is 7 2 x 11?
• Add the two digits together: 7 + 2 = 9.
• Put the answer in between the original digits: 7 2 x 11 = 7 9 2 .
• If the sum is more than 10, place only the final digit and carry the one: 5 7 x 11 = 6 2 7 , because 5 + 7 = 12. The 2 goes in the middle and the 1 gets added to the 5 to make 6.

Turn percentages into easier problems.

• 79% of 10 is the same as 10% of 79. This is true of any two numbers. If you can't find the answer to a percentage problem, try switching it around.
• To find 10% of a number, move the decimal one place to the left (10% of 65 is 6.5). To find 1% of a number, move the decimal two places to the left (1% of 65 is 0.65).
• Use these rules for 10% and 1% to help you with more difficult percentages. For example, 5% is ½ of 10%, so 5% of 80 = (10% of 80) x ½ = 8 x ½ = 4 .
• Break percentages into easier parts: 30% of 900 = (10% of 900) x 3 = 90 x 3 = 270 .

Memorize advanced multiplication shortcuts for specific problems.

• For problems like 84 x 86 , where the tens place is the same and the ones place digits sum to exactly 10, the first digits of the answer are (8 + 1) x 8 = 72 and the last digits are 4 x 6 = 24, for an answer of 7224 . That is, for a problem AB x AC, if B + C = 10, the answer starts with A(A+1) and ends with BC. This also works for larger numbers if all digits besides the ones place are identical. [6] X Research source
• You can rewrite the powers of five (5, 25, 125, 625, ...) as powers of 10 divided by an integer (10 / 2, 100 / 4, 1000 / 8, 10000 / 16, ...). [7] X Research source So 88 x 125 becomes 88 x 1000 ÷ 8 = 88000 ÷ 8 = 11000 .

Memorize squares charts.

• Memorize the squares from 1 to 20 (or higher, if you're ambitious). (That is, 1 x 1 = 1; 2 x 2 = 4; 3 x 3 = 9, and so on.)
• To multiply two numbers, first find their average (the number exactly between them). For example, the average of 18 and 14 is 16.
• Square this answer. Once you've memorized the squares chart, you'll know that 16 x 16 is 256.
• Next, look at the difference between the original numbers and their average: 18 - 16 = 2. (Always use a positive number here.)
• Square this number as well: 2 x 2 = 4.
• To get your final answer, take the first square and subtract the second: 256 - 4 = 252 .

Find useful ways to practice your mental math.

• Flashcards are great for memorizing multiplication and division tables, or for getting used to tricks for specific kinds of problems. Write the problem on one side and the answer on the other, and quiz yourself daily until you get them all right.
• Online math quizzes are another way to test your ability. Look for a well-reviewed app or website made by an educational program.
• Practice in everyday situations. You could add together the total of items you buy as you shop, or multiply the gas cost per volume by your car's tank size to find the total cost. The more of a habit this becomes, the easier it will be.

Joseph Meyer

Exercise your mental math muscles. Improve your math skills by solving daily math problems without using calculators, paper, or counting aids. By solely using your mind and getting into math discussions with your classmates, you will refine your skills and discover new approaches to problem-solving.

Practice Problems and Answers

Community Q&A

• In the real world, you don't always need to know the exact answer. If you're at the grocery store and trying to add 7.07 + 8.95 + 10.09, you could round to the closest whole numbers and estimate that the total is roughly 7 + 9 + 10 = 26. Thanks Helpful 11 Not Helpful 3
• Some people find it easier to think in money than abstract numbers. Instead of 100 - 55, try thinking of a dollar minus a 50¢ coin and a 5¢ coin. Thanks Helpful 6 Not Helpful 8

You Might Also Like

• ↑ http://gizmodo.com/10-tips-to-improve-your-mental-math-ability-1792597814
• ↑ https://www.youtube.com/watch?v=Rgw9Ik5ZGaY
• ↑ https://www.youtube.com/watch?v=SV1dC1KAl_U
• ↑ https://www.youtube.com/watch?v=1JW9BA57aR8
• ↑ http://www.wired.co.uk/article/master-mental-maths
• ↑ https://www.youtube.com/watch?v=YCBTw8KAqkw
• ↑ https://www.scientificamerican.com/article/5-tips-faster-mental-multiplication/
• ↑ Daron Cam. Academic Tutor. Expert Interview. 29 May 2020.

About This Article

One way to improve your mental math skills is to memorize your multiplication and division tables, so you always have the answer to those problems instantly. If you have trouble memorizing the numbers, try creating your own flash cards with blank notecards and asking a friend to help you practice. Another good way to practice your mental math skills is to add up the prices of your items when you’re at the store, and check to make sure you added correctly once the cashier rings you up. You can also try downloading a mental math app like Luminosity to keep your math skills sharp. To learn how to visualize an equation in your head, read on! Did this summary help you? Yes No

• Send fan mail to authors

Reader Success Stories

May 23, 2022

Did this article help you?

Feb 12, 2023

Apr 11, 2023

Ariel Arnaiz

Nov 22, 2022

Deborah Yeary

Jun 8, 2018

Featured Articles

Trending Articles

Watch Articles

• Terms of Use
• Privacy Policy
• Do Not Sell or Share My Info
• Not Selling Info

Don’t miss out! Sign up for

wikiHow’s newsletter

TOP LOCATIONS

• Dallas Fort Worth
• Kansas City
• Long Island
• Los Angeles
• New York City
• Philadelphia
• San Francisco-Bay Area
• Washington DC

Loading Page

HIGH SCHOOL

• ACT Tutoring
• SAT Tutoring
• PSAT Tutoring
• ASPIRE Tutoring
• SHSAT Tutoring
• STAAR Tutoring

GRADUATE SCHOOL

• MCAT Tutoring
• GRE Tutoring
• LSAT Tutoring
• GMAT Tutoring
• AIMS Tutoring
• HSPT Tutoring
• ISAT Tutoring
• SSAT Tutoring

Search 50+ Tests

Math tutoring.

• Elementary Math
• Pre-Calculus
• Trigonometry

science tutoring

Foreign languages.

• Mandarin Chinese

elementary tutoring

• Computer Science

Search 350+ Subjects

• Video Overview
• Tutor Selection Process
• Online Tutoring
• Mobile Tutoring
• Instant Tutoring
• How We Operate
• Our Guarantee
• Impact of Tutoring
• Reviews & Testimonials
• Media Coverage
• About Varsity Tutors

Colleges Decreasing Out-of-State Tuition

Traditionally, out-of-state tuition is much more expensive than in-state, and it is inevitable for students who are interested in out-of-state colleges.

However, some colleges are alleviating or decreasing out-of-state tuition charges, according to The U.S. News and World Report . High-ranking, popular colleges that already attract many intelligent prospective students are not decreasing out-of-state charges. These colleges have many students who are willing to pay the high fees.

Also, these schools have stricter restrictions for who can apply for in-state tuition. Most of these schools only allow students to receive in-state tuition if they graduated from an in-state high school or their parents live in that state, according to The U.S. News and World Report . However, some of these schools are making it easier for out-of-state students to attain in-state tuition.

The U.S. News and World Report stated that there are ways to avoid the higher out-of-state tuition charges by moving to the state to pay taxes and claim residency. However, most students must live in the state for about a year before they can qualify for in-state tuition. Traditionally, the restrictions are tougher in states that attract more students. California is one of the strictest states.

Some colleges are allowing students to register in-state tuition to students after living on campus for a certain amount of time, registering to vote in that state and paying local taxes, according. The restrictions and details are not firm, but if students make efforts toward the aforementioned details, then it will help their cause.

Also, many other colleges reward out-of-state students with in-state tuition if they maintain a certain GPA (usually 2.5 or higher) or achieve a certain ACT/SAT score, according to The U.S. News and World Report .

Many colleges offer in-state tuition to students who live in neighboring states or close to the college, despite it being in a separate state.

You Might Also Enjoy

• Princeton Admissions Information
• Duke University Admissions Information
• Columbia University Admissions Information
• Caltech Admissions Information
• Swarthmore College Admissions Information
• 4 Midterm Mistakes to Avoid
• COVID-19 Updates: List of Standardized Test Changes (Current as of March 20, 2020)
• What is Flow State (and How Can It Help You in School or the Workplace)?
• 3 Bullet Journal Ideas for College Students
• Should I Change My College Major?
• 4 Hacks for an Organized College Life
• View All Past Articles

11 Ways How To Improve Mathematical Problem Solving Skills

• Published August 31, 2022

Introduction

It is crucial to teach individuals how to improve mathematical problem solving skills and for them to take ownership of this skill as they master the subject. There are several reasons why problem-solving is crucial and develops into one of a person’s fundamental talents while addressing math difficulties. 

The capacity to solve problems can be utilized to offer solutions or answers to difficulties addressed more analytically so that someone can be a problem solver. Problem-solving cannot be isolated from daily living. 

To put it another way, when individuals are taught to solve problems, they will be able to make decisions because they have learned how to gather pertinent information, analyze it, and recognize the need to re-examine the outcomes that have been obtained.

Why are Mathematical Problem Solving Skills Important?

Our students must be able to think critically about complex issues because they live in a society that is heavily reliant on information and technology. They must also be able to “analyze and think logically about new situations, devise unspecified solution procedures, and communicate their solution clearly and convincingly to others.” The importance of mathematics education goes beyond the “gatekeeping role that mathematics plays in students’ access to educational and economic possibilities,” as it also prepares pupils for life after school through problem-solving skills and the acquisition of problem-solving strategies.

The idea that mathematics is essentially about reasoning, not memorizing, leads to the significance of problem-solving in math education. Instead of just recalling and using a set of instructions, problem-solving enables students to understand and articulate the steps taken to reach solutions. Students get a deeper comprehension of mathematical ideas, increase their engagement, and recognize the relevance and utility of mathematics through problem-solving. 

Mathematical problem-solving stimulates the development of:

• The capacity for rational, analytical, and innovative thought
• Information processing skills 
• The capacity to order and organize 
• Intellectual challenge enjoyment
• The capacity to solve issues that aid in world exploration and comprehension

To show students the value of mathematics in the world around them, problem-solving should be at the core of all mathematics instruction. With the aid of this approach, students can develop, assess, and improve both their own and other people’s theories about mathematics.

How To Improve Mathematical Problem Solving Skills

The majority of people just require more time and practice to fully learn the subject; they aren’t horrible at math.

What can you do to assist your student to become more skilled at solving mathematical problems? Use our top 11 suggestions to enhance your ability to solve mathematical problems quickly and effectively.

• Read Carefully, Comprehend, And Determine The Nature Of The Problem .

Check the nature of the problem when you first begin studying math to see if it is a word problem, a problem involving fractions, a problem involving quadratic equations, or any other form.

It is crucial to read the problem carefully and make sure you have mastered it before moving on to the next stage.

• Be Able To Comprehend The Ideas

Although repetition and practice are beneficial, it will be challenging to advance if you don’t comprehend the concept.

Fortunately, there are several effective techniques to simplify mathematical ideas. Finding the one that works best for your child is the secret.

For students who are having trouble understanding complex mathematical concepts, arithmetic manipulatives can be a game-changer. Ideas can come to life when arithmetic is taken off the paper and placed in the student’s hands. When you count toy cars or play with blocks, numbers become less ethereal and more tangible. Learning basic math can become more understandable by creating these “sets” of objects.

• Consider Using Games To Learn .

Repetition is crucial for arithmetic preparation, but it may quickly grow tiresome. Nobody likes having to repeatedly copy their times tables. Bring back the excitement if math class has turned into a chore!

An excellent approach to putting new ideas into practice and reinforcing prior knowledge is through game-based learning. Even better, it may add interest and enjoyment to repetition.

Game-based education can take the form of a Friday night family game night or an educational program.

• Include Math In Your Daily Lives.

Every day, you use simple math. Help your student see the math that is there all around them as you go about your day. Determine the savings you’ll experience at your upcoming Target visit.

Determine how many apples you’ll need to purchase at the supermarket.

While baking, clarify that 6 1/4 cups of flour equals 1 cup and a half. Then, enjoy some cookies!

Show your student how math is utilized every day and connect it to something they enjoy doing. It’s not necessary for math to be enigmatic or abstract. Instead, organize tea parties or race monster trucks using math. Dissect it, dispel their apprehension, and watch their interest in arithmetic rise.

• Put Math Into Daily Practice

Math practice is crucial. You must master the mechanics after grasping the notion. And frequently, it’s a practice that makes the idea ultimately make sense. In either case, learning arithmetic involves more than merely memorizing formulas.

It might be challenging to implement the daily practice, especially with a child who dislikes arithmetic. The above-mentioned game-based learning is ideal at this moment. Or choose a game that complements their current lesson. Are they learning about squares? Create them using the math link cubes. Avoid using worksheets and flashcards as much as you can and seek out practice elsewhere.

• Draw Word Puzzles.

Nothing incites anxiety like an unanticipated word puzzle. A math learner who is having trouble can experience a mental breakdown when faced with words and numbers. However, things don’t have to be that way.

Many word puzzles only require step-by-step dissection. Drawing it out is a fantastic technique to accomplish this. How many does Doug have left after eating two of each after having five apples and four oranges? Draw it, discuss it, mark them off, then add up the results.

Many word problems will start to feel familiar if you’ve been walking your student through the numerous math difficulties you run into daily.

• Set Realistic Goals.

Adding more study time will help your student catch up if they are falling behind in math. However, pressuring students to squeeze an extra hour of math into their day is unlikely to result in improved outcomes. Determine their major challenges before expecting any positive changes. Then make practical goals that solve these difficulties.

They will only become more frustrated if they practice a concept for two more hours. Even if they can solve a problem mechanically, the subsequent lesson will leave them feeling just as lost.

Try short practice sessions instead, and enlist some extra assistance. Try a different approach, talk to their teacher, or use an online math tutor. Make sure the additional time is spent on the actual issue and not merely reinforcing the notion that math is difficult and boring.

• Create A Strategy To Address Math Problems.

To create a solution strategy, there are only four easy procedures that must be followed. The procedures are as follows:

Firstly, the formula which will be used to solve the problem must be determined. Here you need to spend some time examining the principles in your textbooks that will help you solve the problem.

• To get the solution to your issue, you must put your needs in writing. To do this, you must create a step-by-step list of the materials you will need to address the issue and maintain organization.
• Tackle the simple problem first. Sometimes the formulas used to solve both problems are redundant. 
• Before solving, try to estimate the solution by making an educated guess about the solution. 
• Review the estimate once more to make sure you didn’t forget anything.
• Consult A Math Tutor

Look into hiring a math tutor if your student is having trouble with big-picture ideas. Because every learner processes information differently, you and your child’s teacher might be missing the “aha” moment that a little extra time and the right tutor can bring.

It’s fantastic when your student finally understands a mathematical concept.   Your student can develop math confidence with the appropriate technique, and who knows, they might even start to enjoy it.

• Concentrate On One Concept At A Time.

Math reinforces itself. Your student cannot skip a lesson and return to it later if they are having difficulty with it at the moment. This is the moment to examine and reinforce the existing thought once more until it makes sense.

Find alternative approaches to new mathematical concepts. Utilize math tools to make numbers visible on a page. Alternatively, try a learning app with enticing prizes and encouraging feedback to promote more practice.

When frustration levels are high, take a step back, but resist the urge to just let it go. Once the idea sinks in, they’ll be eager to move forward.

Teachers need to understand how to develop students’ abilities to improve mathematical problem-solving skills as well as how these abilities grow over time and are considerably enhanced by good teaching methods. 

What teachers know and believe about mathematics and what they comprehend about mathematics teaching and learning greatly influences how they set up classroom instruction. The first step in the teacher’s job is to choose challenging problems that require students to apply their math knowledge. 

In addition to helping students develop their thinking skills, a problem-solving technique offers a framework in which they can master mathematical ideas.

Frequently Asked Questions (FAQs)

Which math teaching model is the most effective.

The “ Problem-solving methodology ” is therefore suggested as the best method for teaching upper primary kids math. It’s a teaching technique where students see a teacher demonstrate things and then build on their understanding through visual analysis.

How Will You Motivate Your Students To Participate In The Math Class Discussion?

Encourage students to take on a difficult assignment by offering positive feedback, plaudits, and compliments. It’s always great to see children have “aha” moments when they notice problems and understand how to solve them.

What Does Mathematical Proof And Motivation Mean?

Motivation, which is Latin for “ check ,” is used to ascertain the veracity of a claim or the accuracy of certain information (in for example an already given proof).

You must independently confirm the validity of the allegation to demonstrate something.

You can also read Best ways on how to improve math skills for Adults.

Table of Contents

More Related Post

How To Apply For Scholarship In USA

Starting College At 23: A Journey of Possibilities and Growth

Starting College at 20: Embracing the Journey of Higher Education

Starting College At 19: A Guide to a Successful Transition

How To Transfer High Schools: 10 Process Guide

What Grade is Prom: Everything You Need to Know

Top categories, useful links, help & support.

© 2022 / Alright Reserved.

Designed by Dotn’More.

The Times of India

9 Proven tips to improve your Math skills

Posted: 7 March 2024 | Last updated: 13 May 2024

Mastering Maths Techniques

Understanding mathematics is crucial for success. Employ a structured approach, writing down solutions step-by-step, using pen and paper, not just drafts. Late-night revisions may impair reasoning. Visualize problems for better comprehension and solutions.

Optimise Your Sleep for Success

Prioritize quality sleep for exam readiness. Adequate rest enhances focus and attention span, bolstering academic performance significantly.

Collaborative Learning Boosts Understanding

Engage with peers to dissect challenging topics, leveraging diverse perspectives and reducing anxiety. Group study fosters deeper comprehension and systematic problem-solving.

Test Your Understanding Regularly

Test your comprehension by attempting assigned exercises after each study session. Review sections covered before quizzes or tests, clarifying unclear concepts and reinforcing learning.

Document Mathematical Procedures

Document procedures while practising, aiding memory retention and streamlining problem-solving. As familiarity with procedures grows, reliance on notes diminishes.

Utilise Formula Sheets Effectively

Leverage provided formula sheets outside exams, integrating them into practice sessions to reinforce application and understanding.

Emphasize Conceptual Understanding over Memorization

Delve into the logic behind formulas for deeper comprehension, fostering creative problem-solving skills and adaptability.

Actively Engage in Learning

Participate actively in learning through note-taking, questioning, and practical application. Actively engaging with material strengthens understanding and retention.

Embrace Mistakes as Learning Opportunities

View mistakes as stepping stones to improvement, analyzing errors to identify areas for growth and seeking assistance when needed. Overcoming mistakes fosters resilience and enhances problem-solving abilities.

More for You

Personality Test: What Your Arm-Crossing Style Reveals About You!

10 Plant based protein sources to build muscle mass without meat

Rishabh Pant suspended: Sourav Ganguly tried blaming Rajasthan Royals, Sanju Samson; this is what BCCI said

Qatar Airways launches world's first AI-powered flight attendant Sama 2.0

DMK Govt Gives Nod To Prosecute Annamalai For Recalling 1956 Remarks Against Annadurai

The greatest Ferraris we’ve ever tested

Richa Chadha On Heeramandi's Drunk Dancing Scene: After 30-40 Takes, I Thought Let Me Have A Quarter | Exclusive

Sticky Cholesterol: 6 simple ways to bring it down

Is the 2025 Subaru Forester a BETTER compact SUV than a Honda CR-V?

7 Realistic Side Hustles to Make $1000 Per Month in 2024

10 banned foods in India & why they're restricted

In pics: Thousands visit Kedarnath as Char Dham Yatra begins; doors of Badrinath temple to open on May 12

iQOO 12 Review: All-around Flagship Android Phone Under Budget? | Full Review

CBSE Class 12 results: PM Modi congratulates students, says ‘immensely proud’

13 fruits that can help lower cholesterol level naturally

The longest-running car nameplates

Virat Kohli Teases Ishant Sharma After No-Look Six; DC Pacer Replies With Epic Celebration - Watch

Rukhmabai Raut: From child bride to India's first divorcee and female doctor

Airport Lounge Access Through Cards Gets Tougher; Here's What Has Changed

Apple iPhone 16 Pro leaks: Display brightness boost and next-gen chipset expected

share this!

April 29, 2024

This article has been reviewed according to Science X's editorial process and policies . Editors have highlighted the following attributes while ensuring the content's credibility:

fact-checked

trusted source

Intervention based on science of reading and math boosts comprehension and word problem-solving skills

by University of Kansas

New research from the University of Kansas has found that an intervention based on the science of reading and math effectively helped English learners boost their comprehension, visualize and synthesize information, and make connections that significantly improved their math performance.

The intervention , performed for 30 minutes twice a week for 10 weeks with 66 third-grade English language learners who displayed math learning difficulties, improved students' performance when compared to students who received general instruction. This indicates that emphasizing cognitive concepts involved in the science of reading and math are key to helping students improve, according to researchers.

"Word problem-solving is influenced by both the science of reading and the science of math. Key components include number sense, decoding, language comprehension and working memory. Utilizing direct and explicit teaching methods enhances understanding and enables students to effectively connect these skills to solve math problems . This integrated approach ensures that students are equipped with necessary tools to navigate both the linguistic and numerical demands of word problems," said Michael Orosco, professor of educational psychology at KU and lead author of the study.

The intervention incorporates comprehension strategy instruction in both reading and math, focusing and decoding, phonological awareness, vocabulary development, inferential thinking, contextualized learning and numeracy.

"It is proving to be one of the most effective evidence-based practices available for this growing population," Orosco said.

The study, co-written with Deborah Reed of the University of Tennessee, was published in the journal Learning Disabilities Research and Practice .

For the research, trained tutors implemented the intervention, developed by Orosco and colleagues based on cognitive and culturally responsive research conducted over a span of 20 years. One example of an intervention session tested in the study included a script in which a tutor examined a word problem explaining that a person made a quesadilla for his friend Mario and gave him one-fourth of it, then asked students to determine how much remained.

The tutor first asked students if they remembered a class session in which they made quesadillas and what shape they were, and demonstrated concepts by drawing a circle on the board, dividing it into four equal pieces, having students repeat terms like numerator and denominator. The tutor explains that when a question asks how much is left, subtraction is required. The students also collaborated with peers to practice using important vocabulary in sentences. The approach both helps students learn and understand mathematical concepts while being culturally responsive.

"Word problems are complex because they require translating words into mathematical equations, and this involves integrating the science of reading and math through language concepts and differentiated instruction," Orosco said. "We have not extensively tested these approaches with this group of children. However, we are establishing an evidence-based framework that aids them in developing background knowledge and connecting it to their cultural contexts."

Orosco, director of KU's Center for Culturally Responsive Educational Neuroscience, emphasized the critical role of language in word problems, highlighting the importance of using culturally familiar terms. For instance, substituting "pastry" for "quesadilla" could significantly affect comprehension for students from diverse backgrounds. Failure to grasp the initial scenario could impede subsequent problem-solving efforts.

The study proved effective in improving students' problem-solving abilities, despite covariates including an individual's basic calculation skills, fluid intelligence and reading comprehension scores. That finding is key, as while ideally all students would begin on equal footing and there would be few variations in a classroom, in reality, covariates exist and are commonplace.

The study had trained tutors deliver the intervention, and its effectiveness should be further tested with working teachers, the authors wrote. Orosco said professional development to help teachers gain the skills is necessary, and it is vital for teacher preparation programs to train future teachers with such skills as well. And helping students at the elementary level is necessary to help ensure success in future higher-level math classes such as algebra.

The research builds on Orosco and colleagues' work in understanding and improving math instruction for English learners. Future work will continue to examine the role of cognitive functions such as working memory and brain science, as well as potential integration of artificial intelligence in teaching math.

"Comprehension strategy instruction helps students make connections, ask questions, visualize, synthesize and monitor their thinking about word problems," Orosco and Reed wrote. "Finally, applying comprehension strategy instruction supports ELs in integrating their reading, language and math cognition…. Focusing on relevant language in word problems and providing collaborative support significantly improved students' solution accuracy."

Provided by University of Kansas

Explore further

Feedback to editors

Biohybrid robot made from flour and oats could act as a biodegradable vector for reforestation

Island birds more adaptable than previously thought

Scientists discover 'weird' statistics of electrons ejected by intense quantum light

New gel breaks down alcohol in the body

Discovery of biomarkers in space—conditions on Saturn's moon Enceladus simulated in the laboratory

Researchers uncover mechanism for short-distance vesicle movements

Two-year study shows some varieties of annual flowers have a place in pollinator-friendly gardens

The secret to mimicking natural faults? Plexiglass and Teflon

2 hours ago

Indian Ocean sea-surface temperatures found to be accurate predictor of dengue outbreaks

Long-term study finds organic farming leads to adaptations in the genetic material in plants

Relevant physicsforums posts, physics education is 60 years out of date, plagiarism & chatgpt: is cheating with ai the new normal.

3 hours ago

Physics Instructor Minimum Education to Teach Community College

May 11, 2024

Studying "Useful" vs. "Useless" Stuff in School

Apr 30, 2024

Why are Physicists so informal with mathematics?

Apr 29, 2024

Digital oscilloscope for high school use

Apr 25, 2024

More from STEM Educators and Teaching

Related Stories

Study shows program improves teaching skills, students' word problem–solving

Jun 14, 2022

Study shows approach can help English learners improve at math word problems

Jun 19, 2018

Study examines role of working memory, cognitive functions in English learners learning to write

Oct 17, 2023

Cognitive study shows lack of bilingual education adversely affects English language learners' writing skills

Oct 14, 2021

How vocabulary breadth and depth influence bilingual reading comprehension

Aug 21, 2023

Study validates the simple view of reading for enhancing second and foreign language learners' experience

Aug 17, 2023

Recommended for you

Investigation reveals varied impact of preschool programs on long-term school success

May 2, 2024

Training of brain processes makes reading more efficient

Apr 18, 2024

Researchers find lower grades given to students with surnames that come later in alphabetical order

Apr 17, 2024

Earth, the sun and a bike wheel: Why your high-school textbook was wrong about the shape of Earth's orbit

Apr 8, 2024

Touchibo, a robot that fosters inclusion in education through touch

Apr 5, 2024

More than money, family and community bonds prep teens for college success: Study

Let us know if there is a problem with our content.

Use this form if you have come across a typo, inaccuracy or would like to send an edit request for the content on this page. For general inquiries, please use our contact form . For general feedback, use the public comments section below (please adhere to guidelines ).

Please select the most appropriate category to facilitate processing of your request

Thank you for taking time to provide your feedback to the editors.

Your feedback is important to us. However, we do not guarantee individual replies due to the high volume of messages.

E-mail the story

Your email address is used only to let the recipient know who sent the email. Neither your address nor the recipient's address will be used for any other purpose. The information you enter will appear in your e-mail message and is not retained by Phys.org in any form.

Newsletter sign up

Get weekly and/or daily updates delivered to your inbox. You can unsubscribe at any time and we'll never share your details to third parties.

More information Privacy policy

Donate and enjoy an ad-free experience

We keep our content available to everyone. Consider supporting Science X's mission by getting a premium account.

E-mail newsletter

Math Riddles: Boost Your Brain 4+

40 more fun iq-enhancing games, thiem nguyen, designed for ipad, screenshots, description.

Math Riddles: Boost Your Brainpower with Fun IQ-Enhancing Games! Welcome to Math Riddle, the ultimate brain workout game designed to elevate your cognitive abilities and keep your mind sharp! With 40+ unique math-based riddles and observation puzzles, challenge your memory, focus, and problem-solving skills. Whether you're looking to improve your IQ or simply have fun, Math Riddle offers an easy-to-use interface and multiple difficulty levels to keep you engaged. Play anytime, anywhere, as the game is fully offline playable. Get ready for an intriguing mental journey that becomes more captivating with each level!

Version 2.3

- Changed some images for better display quality. - Fix some spelling errors in the text - Performance improvements

App Privacy

The developer, Thiem Nguyen , indicated that the app’s privacy practices may include handling of data as described below. For more information, see the developer’s privacy policy .

Data Not Collected

The developer does not collect any data from this app.

Privacy practices may vary, for example, based on the features you use or your age. Learn More

Information

• Developer Website
• App Support
• Privacy Policy

More By This Developer

Pomodoro: Timer & WebHook

Four Pics Baybayin: Puzzle

Four Corners: Play Together

Mathematics: Quiz & PDF

Math Multiply: Quiz

Sound Trainer: Listen Better

You Might Also Like

Guess The Movie | Film Quiz

Christmas Spirit: Grimm Tales

Bitter Awakening F2P

Dark City: Intrigue

Puzzle Packed IQ Games

Math | Riddles and Puzzles