de broglie hypothesis class 12 derivation

Talk to our experts

1800-120-456-456

• De Broglie Equation

Introduction

The wave nature of light was the only aspect that was considered until Neil Bohr’s model. Later, however, Max Planck in his explanation of quantum theory hypothesized that light is made of very minute pockets of energy which are in turn made of photons or quanta. It was then considered that light has a particle nature and every packet of light always emits a certain fixed amount of energy. 

By this, the energy of photons can be expressed as:

E = hf = h * c/λ

Here, h is Plank’s constant

F refers to the frequency of the waves

Λ implies the wavelength of the pockets

Therefore, this basically insinuates that light has both the properties of particle duality as well as wave. 

Louis de Broglie was a student of Bohr, who then formulated his own hypothesis of wave-particle duality, drawn from this understanding of light. Later on, when this hypothesis was proven true, it became a very important concept in particle physics. 

⇒ Don't Miss Out: Get Your Free JEE Main Rank Predictor 2024 Instantly! 🚀

What is the De Broglie Equation?

Quantum mechanics assumes matter to be both like a wave as well as a particle at the sub-atomic level. The De Broglie equation states that every particle that moves can sometimes act as a wave, and sometimes as a particle. The wave which is associated with the particles that are moving are known as the matter-wave, and also as the De Broglie wave. The wavelength is known as the de Broglie wavelength. 

For an electron, de Broglie wavelength equation is:       

λ = \[\frac{h}{mv}\]

Here, λ points to the wave of the electron in question

M is the mass of the electron

V is the velocity of the electron

Mv is the momentum that is formed as a result

It was found out that this equation works and applies to every form of matter in the universe, i.e, Everything in this universe, from living beings to inanimate objects, all have wave particle duality. 

Significance of De Broglie Equation

De Broglie says that all the objects that are in motion have a particle nature. However, if we look at a moving ball or a moving car, they don’t seem to have particle nature. To make this clear, De Broglie derived the wavelengths of electrons and a cricket ball. Now, let’s understand how he did this.  

De Broglie Wavelength 

1. De Broglie Wavelength for a Cricket Ball

Let’s say,Mass of the ball  = 150 g (150 x 10⁻³ kg),

Velocity = 35 m/s, 

and  h = 6.626 x 10⁻³⁴ Js

Now, putting these values in the equation 

λ = (6.626 * 10 to power of -34)/ (150 * 10 to power of -3 *35) 

This yields

λBALL = 1.2621 x 10 to the power of -34 m,

Which is 1.2621 x 10 to the power of -24 Å.

We know that Å is a very small unit, and therefore the value is in the power of 10−24−24^{-24}, which is a very small value. From here, we see that the moving cricket ball is a particle.

Now, the question arises if this ball has a wave nature or not. Your answer will be a big no because the value of λBALL is immeasurable. This proves that de Broglie’s theory of wave-particle duality is valid for the moving objects ‘up to’ the size (not equal to the size) of the electrons.

De Broglie Wavelength for an Electron

We know that me  = 9.1 x 10 to power of -31 kg

and ve = 218 x 10 to power of -6 m/s

Now, putting these values in the equation  λ = h/mv, which yields λ = 3.2 Å. 

This value is measurable. Therefore, we can say that electrons have wave-particle duality. Thus all the big objects have a wave nature and microscopic objects like electrons have wave-particle nature.

E  = hν  = \[\frac{hc}{\lambda }\]

The Conclusion of De Broglie Hypothesis

From de Broglie equation for a material particle, i.e.,  

λ = \[\frac{h}{p}\]or \[\frac{h}{mv}\], we conclude the following:

i. If v = 0, then λ = ∞, and

If v = ∞, then λ = 0

It means that waves are associated with the moving material particles only. This implies these waves are independent of their charge. 

FAQs on De Broglie Equation

1.The De Broglie hypothesis was confirmed through which means?

De Broglie had not proved the validity of his hypothesis on his own, it was merely a hypothetical assumption before it was tested out and consequently, it was found that all substances in the universe have wave-particle duality. A number of experiments were conducted with Fresnel diffraction as well as a specular reflection of neutral atoms. These experiments proved the validity of De Broglie’s statements and made his hypothesis come true. These experiments were conducted by some of his students. 

2.What exactly does the De Broglie equation apply to?

In very broad terms, this applies to pretty much everything in the tangible universe. This means that people, non-living things, trees and animals, all of these come under the purview of the hypothesis. Any particle of any substance that has matter and has linear momentum also is a wave. The wavelength will be inversely related to the magnitude of the linear momentum of the particle. Therefore, everything in the universe that has matter, is applicable to fit under the De Broglie equation. 

3.Is it possible that a single photon also has a wavelength?

When De Broglie had proposed his hypothesis, he derived from the work of Planck that light is made up of small pockets that have a certain energy, known as photons. For his own hypothesis, he said that all things in the universe that have to matter have wave-particle duality, and therefore, wavelength. This extends to light as well, since it was proved that light is made up of matter (photons). Hence, it is true that even a single photon has a wavelength. 

4.Are there any practical applications of the De Broglie equation?

It would be wrong to say that people use this equation in their everyday lives, because they do not, not in the literal sense at least. However, practical applications do not only refer to whether they can tangibly be used by everyone. The truth of the De Broglie equation lies in the fact that we, as human beings, also are made of matter and thus we also have wave-particle duality. All the things we work with have wave-particle duality. 

5.Does the De Broglie equation apply to an electron?

Yes, this equation is applicable for every single moving body in the universe, down to the smallest subatomic levels. Just how light particles like photons have their own wavelengths, it is also true for an electron. The equation treats electrons as both waves as well as particles, only then will it have wave-particle duality. For every electron of every atom of every element, this stands true and using the equation mentioned, the wavelength of an electron can also be calculated.  

6.Derive the relation between De Broglie wavelength and temperature.

We know that the average KE of a particle is:

                       K = 3/2 k b T

Where k b is Boltzmann’s constant, and

T   = temperature in Kelvin

The kinetic energy of a particle is  ½ mv²

The momentum of a particle, p = mv = √2mK

= √2m(3/2)KbT = √2mKbT 

de Broglie wavelength, λ = h/p = h√2mkbT 

7.If an electron behaves like a wave, what should determine its wavelength and frequency?

Momentum and energy determine the wavelength and frequency of an electron.

8. Find λ associated with an H 2 of mass 3 a.m.u moving with a velocity of 4 km/s.

Here,  v = 4 x 10³ m/s 

Mass of hydrogen = 3 a.m.u = 3 x 1.67 x 10⁻²⁷kg = 5 x 10⁻²⁷kg    

On putting these values in the equation λ = h/mv we get

λ = (6.626 x 10⁻³⁴)/(4 x 10³ x 5 x 10⁻²⁷) = 3 x 10⁻¹¹ m.

9. If the KE of an electron increases by 21%, find the percentage change in its De Broglie wavelength.

We know that  λ = h/√2mK

So,  λ i = h/√(2m x 100) , and λ f = h/√(2m x 121)

% change in λ is:

Change in wavelength/Original x 100 = (λ fi - λ f )/λ i = ((h/√2m)(1/10 - 1/21))/(h/√2m)(1/10) 

On solving, we get

% change in λ = 5.238 %

• Wave Nature of Matter

What does wave nature of matter mean? Can a small particle be at multiple places at the same time? Do I have a wave nature? Why can’t I see it? Let’s try to answer these questions.

Suggested Videos

In the earlier articles, we saw how light behaves both as a wave and particle. A particle is confined at a place. On the other hand, a wave is spread in space. We say that the nature of light depends on the nature of our observation. If you are observing phenomenon like the interference, diffraction or reflection, you will find that light is a wave. However, if you are looking at phenomena like the photoelectric effect, you will find that light has a particle character.

You might ask, which is it? Is light a wave or a particle? The answer is that it has a dual nature. You may also wonder whether it is a specific property of light! Does only light have a dual nature? What if other quantities had dual nature? How could we measure and prove that? Maybe these were the questions that led Louis Victor de Broglie to come up with one of the most revolutionary equations in Physics, the de Broglie equation.

Browse more Topics under Dual Nature Of Radiation And Matter

• Electron Emission
• Experimental Study of Photoelectric Effect
• Davisson and Germer Experiment
• Einstein’s Photoelectric Equation: Energy Quantum of Radiation

Next up – A Few Lines of Math That Go A Long Way!

Let us recall the mass-energy equivalence of Einstein, E =mc 2 …(1)

Also from Einstein-Plank relation, we have: E = hν …(2)

Furthermore, we see that equation (1) is applicable to particles with some “mass”. In other words equation (1) can be applied to particles and equation (2) is an equation for a wave of frequency ν. So the two were not equated until de Broglie had a breakthrough! We know that light can be a wave as well as a particle. In that case, we can say that equation (1) and (2)  represent the same quantity. Consequently, we must have: hν = mc 2 . Since we know that ν = c/λ, we have:

h(c/λ) =  mc 2

λ = h/mc; where ‘c’ is the velocity of light. If we have a wave of velocity, say ‘v’, we can write: λ = h/mv

or λ = h/p   …(3)

where ‘p’ is the momentum of the wave-particle! See what we did here? We have mass – a particle property, in the same equation as wavelength – a wave property. Thus if matter exhibits wave properties, it must be given by equation (3). Equation (3) is the de Broglie equation and represents the wave-particle duality. Hence we say that everything in the Cosmos exhibits a dual nature. This is the wave nature of radiation and matter.

Wavelength of Macroscopic Objects

“So you are telling me that I am not a particle but a wave? Where is it then?” First of all, you are both. Let us find out your wavelength. Suppose you have a mass of 55 kg. If you are at rest i.e. if the velocity = 0, then we see from equation (3), that λ is not defined. So not much help there! Let us say that you are moving at a velocity of 5 m/s. Using equation (3), we can see that

λ = h/(55)×5

λ = 6.63×10-34/275  ≈ 2.4×10-36 m

As you can see, you can’t “see” this small wavelength. Thus the wavelength of macroscopic objects is too small to have any observable effects on any property at normal velocities.

Learn more about Wave Optics .

So de Broglie Guessed An Equation And Everyone Just Agreed?

Fortunately, there was a way to verify this equation. Let us see the equation again, λ = h/p

We know that K.E. = 1/2(mv 2 )

or K.E. = \( \frac{(mv)^2}{(2m)} \) = \( \frac{(p)^2}{(2m)} \)

Here, p is the momentum. Thus we have: p = \( \sqrt[]{2mE} \)  …. (4)

Using (4) in (3), we have: λ = h/\( \sqrt[]{2mE} \) …(5)

Also for a charged particle, E = eV and we have: λ = h/\( \sqrt[]{2meV} \)

So for an electron e = 1.6×10 -19 C and m = 9.10938356 × 10 -31 kilograms, we have:

Hence we can verify the de Broglie equation if we observe the motion of an electron. This was done in the Davisson and Germer Experiment.

Solved Examples For You

The de-Broglie wavelength of an electron (mass 1 × 10 − 30 k g , charge = 1.6 × 10 − 19   C ) with a kinetic energy of 200 e V is: (Planck’s constant 6.6 × 10 − 34 J ):

A) 9.60 × 10 − 11 m                                             B) 8.25 × 10 − 11 m

C) 6.25 × 10 − 11 m                                             C) 5.00 × 10 − 11 m

Solution: B) 8.25 × 10 − 11 m

We can directly use equation (5) i.e. λ = h/\( \sqrt[]{2mE} \). Substitution of the respective values gives the required result.

Customize your course in 30 seconds

Which class are you in.

Dual Nature of Radiation and Matter

• Radiation Detector
• Cherenkov Radiation
• Einstein’s Photoelectric Equation: Energy Quantum of Radiation

One response to “Davisson and Germer Experiment”

Eassy understand

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

Download the App

Reset password New user? Sign up

Existing user? Log in

De Broglie Hypothesis

Already have an account? Log in here.

Today we know that every particle exhibits both matter and wave nature. This is called wave-particle duality . The concept that matter behaves like wave is called the de Broglie hypothesis , named after Louis de Broglie, who proposed it in 1924.

De Broglie Equation

Explanation of bohr's quantization rule.

De Broglie gave the following equation which can be used to calculate de Broglie wavelength, \(\lambda\), of any massed particle whose momentum is known:

\[\lambda = \frac{h}{p},\]

where \(h\) is the Plank's constant and \(p\) is the momentum of the particle whose wavelength we need to find.

With some modifications the following equation can also be written for velocity \((v)\) or kinetic energy \((K)\) of the particle (of mass \(m\)):

\[\lambda = \frac{h}{mv} = \frac{h}{\sqrt{2mK}}.\]

Notice that for heavy particles, the de Broglie wavelength is very small, in fact negligible. Hence, we can conclude that though heavy particles do exhibit wave nature, it can be neglected as it's insignificant in all practical terms of use.

Calculate the de Broglie wavelength of a golf ball whose mass is 40 grams and whose velocity is 6 m/s. We have \[\lambda = \frac{h}{mv} = \frac{6.63 \times 10^{-34}}{40 \times 10^{-3} \times 6} \text{ m}=2.76 \times 10^{-33} \text{ m}.\ _\square\]

One of the main limitations of Bohr's atomic theory was that no justification was given for the principle of quantization of angular momentum. It does not explain the assumption that why an electron can rotate only in those orbits in which the angular momentum of the electron, \(mvr,\) is a whole number multiple of \( \frac{h}{2\pi} \).

De Broglie successfully provided the explanation to Bohr's assumption by his hypothesis.

Problem Loading...

Note Loading...

Set Loading...

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

6.6: De Broglie’s Matter Waves

• Last updated
• Save as PDF
• Page ID 4524

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Learning Objectives

By the end of this section, you will be able to:

• Describe de Broglie’s hypothesis of matter waves
• Explain how the de Broglie’s hypothesis gives the rationale for the quantization of angular momentum in Bohr’s quantum theory of the hydrogen atom
• Describe the Davisson–Germer experiment
• Interpret de Broglie’s idea of matter waves and how they account for electron diffraction phenomena

Compton’s formula established that an electromagnetic wave can behave like a particle of light when interacting with matter. In 1924, Louis de Broglie proposed a new speculative hypothesis that electrons and other particles of matter can behave like waves. Today, this idea is known as de Broglie’s hypothesis of matter waves . In 1926, De Broglie’s hypothesis, together with Bohr’s early quantum theory, led to the development of a new theory of wave quantum mechanics to describe the physics of atoms and subatomic particles. Quantum mechanics has paved the way for new engineering inventions and technologies, such as the laser and magnetic resonance imaging (MRI). These new technologies drive discoveries in other sciences such as biology and chemistry.

According to de Broglie’s hypothesis, massless photons as well as massive particles must satisfy one common set of relations that connect the energy \(E\) with the frequency \(f\), and the linear momentum \(p\) with the wavelength \(λ\). We have discussed these relations for photons in the context of Compton’s effect. We are recalling them now in a more general context. Any particle that has energy and momentum is a de Broglie wave of frequency \(f\) and wavelength \(\lambda\):

\[ E = h f \label{6.53} \]

\[ \lambda = \frac{h}{p} \label{6.54} \]

Here, \(E\) and \(p\) are, respectively, the relativistic energy and the momentum of a particle. De Broglie’s relations are usually expressed in terms of the wave vector \(\vec{k}\), \(k = 2 \pi / \lambda\), and the wave frequency \(\omega = 2 \pi f\), as we usually do for waves:

\begin{aligned} &E=\hbar \omega \label{6.55}\\ &\vec{p}=\hbar \vec{k} \label{6.56} \end{aligned}

Wave theory tells us that a wave carries its energy with the group velocity . For matter waves, this group velocity is the velocity \(u\) of the particle. Identifying the energy E and momentum p of a particle with its relativistic energy \(mc^2\) and its relativistic momentum \(mu\), respectively, it follows from de Broglie relations that matter waves satisfy the following relation:

\[ \lambda f =\frac{\omega}{k}=\frac{E / \hbar}{p / \hbar}=\frac{E}{p} = \frac{m c^{2}}{m u}=\frac{c^{2}}{u}=\frac{c}{\beta} \label{6.57} \]

where \(\beta = u/c\). When a particle is massless we have \(u=c\) and Equation \ref{6.57} becomes \(\lambda f = c\).

Example \(\PageIndex{1}\): How Long are de Broglie Matter Waves?

Calculate the de Broglie wavelength of:

• a 0.65-kg basketball thrown at a speed of 10 m/s,
• a nonrelativistic electron with a kinetic energy of 1.0 eV, and
• a relativistic electron with a kinetic energy of 108 keV.

We use Equation \ref{6.57} to find the de Broglie wavelength. When the problem involves a nonrelativistic object moving with a nonrelativistic speed u , such as in (a) when \(\beta=u / c \ll 1\), we use nonrelativistic momentum p . When the nonrelativistic approximation cannot be used, such as in (c), we must use the relativistic momentum \(p=m u=m_{0} \gamma u=E_{0} \gamma \beta/c\), where the rest mass energy of a particle is \(E_0 = m c^2 \) and \(\gamma\) is the Lorentz factor \(\gamma=1 / \sqrt{1-\beta^{2}}\). The total energy \(E\) of a particle is given by Equation \ref{6.53} and the kinetic energy is \(K=E-E_{0}=(\gamma-1) E_{0}\). When the kinetic energy is known, we can invert Equation 6.4.2 to find the momentum

\[ p=\sqrt{\left(E^{2}-E_{0}^{2}\right) / c^{2}}=\sqrt{K\left(K+2 E_{0}\right)} / c \nonumber \]

and substitute into Equation \ref{6.57} to obtain

\[ \lambda=\frac{h}{p}=\frac{h c}{\sqrt{K\left(K+2 E_{0}\right)}} \label{6.58} \]

Depending on the problem at hand, in this equation we can use the following values for hc :

\[ h c=\left(6.626 \times 10^{-34} \: \mathrm{J} \cdot \mathrm{s}\right)\left(2.998 \times 10^{8} \: \mathrm{m} / \mathrm{s}\right)=1.986 \times 10^{-25} \: \mathrm{J} \cdot \mathrm{m}=1.241 \: \mathrm{eV} \cdot \mu \mathrm{m} \nonumber \]

• For the basketball, the kinetic energy is \[ K=m u^{2} / 2=(0.65 \: \mathrm{kg})(10 \: \mathrm{m} / \mathrm{s})^{2} / 2=32.5 \: \mathrm{J} \nonumber \] and the rest mass energy is \[ E_{0}=m c^{2}=(0.65 \: \mathrm{kg})\left(2.998 \times 10^{8} \: \mathrm{m} / \mathrm{s}\right)^{2}=5.84 \times 10^{16} \: \mathrm{J} \nonumber \] We see that \(K /\left(K+E_{0}\right) \ll 1\) and use \(p=m u=(0.65 \: \mathrm{kg})(10 \: \mathrm{m} / \mathrm{s})=6.5 \: \mathrm{J} \cdot \mathrm{s} / \mathrm{m} \): \[ \lambda=\frac{h}{p}=\frac{6.626 \times 10^{-34} \: \mathrm{J} \cdot \mathrm{s}}{6.5 \: \mathrm{J} \cdot \mathrm{s} / \mathrm{m}}=1.02 \times 10^{-34} \: \mathrm{m} \nonumber \]
• For the nonrelativistic electron, \[ E_{0}=mc^{2}=\left(9.109 \times 10^{-31} \mathrm{kg}\right)\left(2.998 \times 10^{8} \mathrm{m} / \mathrm{s}\right)^{2}=511 \mathrm{keV} \nonumber \] and when \(K = 1.0 \: eV\), we have \(K/(K+E_0) = (1/512) \times 10^{-3} \ll 1\), so we can use the nonrelativistic formula. However, it is simpler here to use Equation \ref{6.58}: \[ \lambda=\frac{h}{p}=\frac{h c}{\sqrt{K\left(K+2 E_{0}\right)}}=\frac{1.241 \: \mathrm{eV} \cdot \mu \mathrm{m}}{\sqrt{(1.0 \: \mathrm{eV})[1.0 \: \mathrm{eV}+2(511 \: \mathrm{keV})]}}=1.23 \: \mathrm{nm} \nonumber \] If we use nonrelativistic momentum, we obtain the same result because 1 eV is much smaller than the rest mass of the electron.
• For a fast electron with \(K=108 \: keV\), relativistic effects cannot be neglected because its total energy is \(E = K = E_0 = 108 \: keV + 511 \: keV = 619 \: keV\) and \(K/E = 108/619\) is not negligible: \[ \lambda=\frac{h}{p}=\frac{h c}{\sqrt{K\left(K+2 E_{0}\right)}}=\frac{1.241 \: \mathrm{eV} \cdot \mu \mathrm{m}}{\sqrt{108 \: \mathrm{keV}[108 \: \mathrm{keV}+2(511 \: \mathrm{keV})]}}=3.55 \: \mathrm{pm} \nonumber \].

Significance

We see from these estimates that De Broglie’s wavelengths of macroscopic objects such as a ball are immeasurably small. Therefore, even if they exist, they are not detectable and do not affect the motion of macroscopic objects.

Exercise \(\PageIndex{1}\)

What is de Broglie’s wavelength of a nonrelativistic proton with a kinetic energy of 1.0 eV?

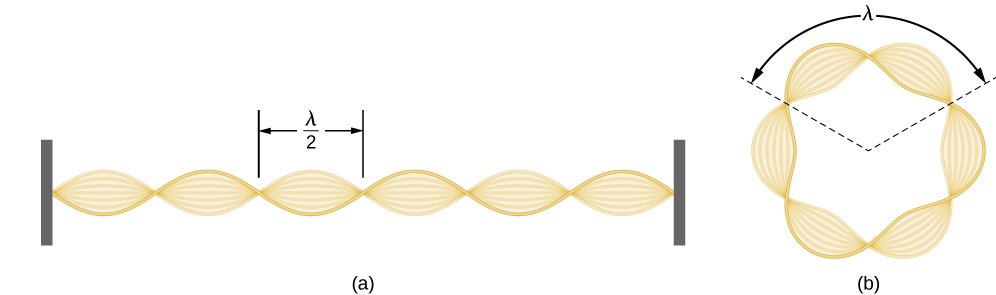
Using the concept of the electron matter wave, de Broglie provided a rationale for the quantization of the electron’s angular momentum in the hydrogen atom, which was postulated in Bohr’s quantum theory. The physical explanation for the first Bohr quantization condition comes naturally when we assume that an electron in a hydrogen atom behaves not like a particle but like a wave. To see it clearly, imagine a stretched guitar string that is clamped at both ends and vibrates in one of its normal modes. If the length of the string is l (Figure \(\PageIndex{1}\)), the wavelengths of these vibrations cannot be arbitrary but must be such that an integer k number of half-wavelengths \(\lambda/2\) fit exactly on the distance l between the ends. This is the condition \(l=k \lambda /2\) for a standing wave on a string. Now suppose that instead of having the string clamped at the walls, we bend its length into a circle and fasten its ends to each other. This produces a circular string that vibrates in normal modes, satisfying the same standing-wave condition, but the number of half-wavelengths must now be an even number \(k\), \(k=2n\), and the length l is now connected to the radius \(r_n\) of the circle. This means that the radii are not arbitrary but must satisfy the following standing-wave condition:

\[ 2 \pi r_{n}=2 n \frac{\lambda}{2} \label{6.59}. \]

If an electron in the n th Bohr orbit moves as a wave, by Equation \ref{6.59} its wavelength must be equal to \(\lambda = 2 \pi r_n / n\). Assuming that Equation \ref{6.58} is valid, the electron wave of this wavelength corresponds to the electron’s linear momentum, \(p = h/\lambda = nh / (2 \pi r_n) = n \hbar /r_n\). In a circular orbit, therefore, the electron’s angular momentum must be

\[ L_{n}=r_{n} p=r_{n} \frac{n \hbar}{r_{n}}=n \hbar \label{6.60} . \]

This equation is the first of Bohr’s quantization conditions, given by Equation 6.5.6 . Providing a physical explanation for Bohr’s quantization condition is a convincing theoretical argument for the existence of matter waves.

Example \(\PageIndex{2}\): The Electron Wave in the Ground State of Hydrogen

Find the de Broglie wavelength of an electron in the ground state of hydrogen.

We combine the first quantization condition in Equation \ref{6.60} with Equation 6.5.6 and use Equation 6.5.9 for the first Bohr radius with \(n = 1\).

When \(n=1\) and \(r_n = a_0 = 0.529 \: Å\), the Bohr quantization condition gives \(a_{0} p=1 \cdot \hbar \Rightarrow p=\hbar / a_{0}\). The electron wavelength is:

\[ \lambda=h / p = h / \hbar / a_{0} = 2 \pi a_{0} = 2 \pi(0.529 \: Å)=3.324 \: Å .\nonumber \]

We obtain the same result when we use Equation \ref{6.58} directly.

Exercise \(\PageIndex{2}\)

Find the de Broglie wavelength of an electron in the third excited state of hydrogen.

\(\lambda = 2 \pi n a_0 = 2 (3.324 \: Å) = 6.648 \: Å\)

Experimental confirmation of matter waves came in 1927 when C. Davisson and L. Germer performed a series of electron-scattering experiments that clearly showed that electrons do behave like waves. Davisson and Germer did not set up their experiment to confirm de Broglie’s hypothesis: The confirmation came as a byproduct of their routine experimental studies of metal surfaces under electron bombardment.

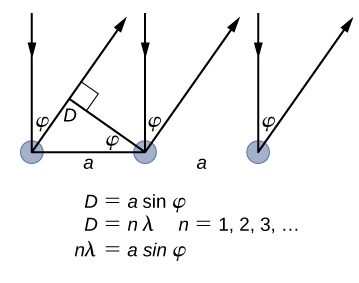
In the particular experiment that provided the very first evidence of electron waves (known today as the Davisson–Germer experiment ), they studied a surface of nickel. Their nickel sample was specially prepared in a high-temperature oven to change its usual polycrystalline structure to a form in which large single-crystal domains occupy the volume. Figure \(\PageIndex{2}\) shows the experimental setup. Thermal electrons are released from a heated element (usually made of tungsten) in the electron gun and accelerated through a potential difference ΔV, becoming a well-collimated beam of electrons produced by an electron gun. The kinetic energy \(K\) of the electrons is adjusted by selecting a value of the potential difference in the electron gun. This produces a beam of electrons with a set value of linear momentum, in accordance with the conservation of energy:

\[ e \Delta V=K=\frac{p^{2}}{2 m} \Rightarrow p=\sqrt{2 m e \Delta V} \label{6.61} \]

The electron beam is incident on the nickel sample in the direction normal to its surface. At the surface, it scatters in various directions. The intensity of the beam scattered in a selected direction φφ is measured by a highly sensitive detector. The detector’s angular position with respect to the direction of the incident beam can be varied from φ=0° to φ=90°. The entire setup is enclosed in a vacuum chamber to prevent electron collisions with air molecules, as such thermal collisions would change the electrons’ kinetic energy and are not desirable.

When the nickel target has a polycrystalline form with many randomly oriented microscopic crystals, the incident electrons scatter off its surface in various random directions. As a result, the intensity of the scattered electron beam is much the same in any direction, resembling a diffuse reflection of light from a porous surface. However, when the nickel target has a regular crystalline structure, the intensity of the scattered electron beam shows a clear maximum at a specific angle and the results show a clear diffraction pattern (see Figure \(\PageIndex{3}\)). Similar diffraction patterns formed by X-rays scattered by various crystalline solids were studied in 1912 by father-and-son physicists William H. Bragg and William L. Bragg. The Bragg law in X-ray crystallography provides a connection between the wavelength \(\lambda\) of the radiation incident on a crystalline lattice, the lattice spacing, and the position of the interference maximum in the diffracted radiation (see Diffraction ).

The lattice spacing of the Davisson–Germer target, determined with X-ray crystallography, was measured to be \(a=2.15 \: Å\). Unlike X-ray crystallography in which X-rays penetrate the sample, in the original Davisson–Germer experiment, only the surface atoms interact with the incident electron beam. For the surface diffraction, the maximum intensity of the reflected electron beam is observed for scattering angles that satisfy the condition nλ = a sin φ (see Figure \(\PageIndex{4}\)). The first-order maximum (for n=1) is measured at a scattering angle of φ≈50° at ΔV≈54 V, which gives the wavelength of the incident radiation as λ=(2.15 Å) sin 50° = 1.64 Å. On the other hand, a 54-V potential accelerates the incident electrons to kinetic energies of K = 54 eV. Their momentum, calculated from Equation \ref{6.61}, is \(p = 2.478 \times 10^{−5} \: eV \cdot s/m\). When we substitute this result in Equation \ref{6.58}, the de Broglie wavelength is obtained as

\[ \lambda=\frac{h}{p}=\frac{4.136 \times 10^{-15} \mathrm{eV} \cdot \mathrm{s}}{2.478 \times 10^{-5} \mathrm{eV} \cdot \mathrm{s} / \mathrm{m}}=1.67 \mathrm{Å} \label{6.62}. \]

The same result is obtained when we use K = 54eV in Equation \ref{6.61}. The proximity of this theoretical result to the Davisson–Germer experimental value of λ = 1.64 Å is a convincing argument for the existence of de Broglie matter waves.

Diffraction lines measured with low-energy electrons, such as those used in the Davisson–Germer experiment, are quite broad (Figure \(\PageIndex{3}\)) because the incident electrons are scattered only from the surface. The resolution of diffraction images greatly improves when a higher-energy electron beam passes through a thin metal foil. This occurs because the diffraction image is created by scattering off many crystalline planes inside the volume, and the maxima produced in scattering at Bragg angles are sharp (Figure \(\PageIndex{5}\)).

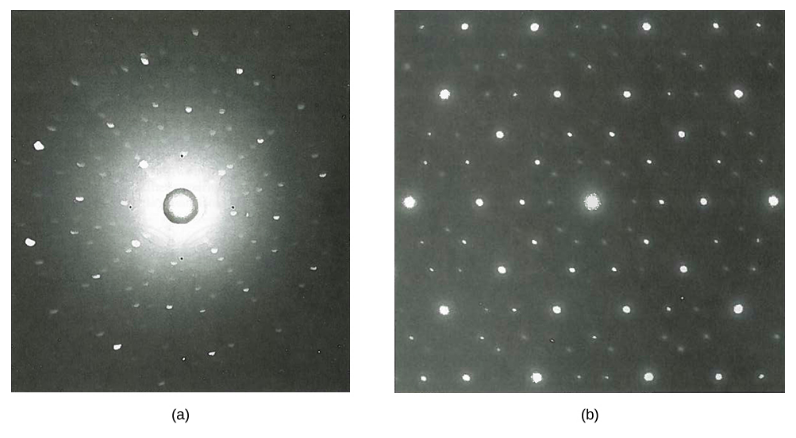
Since the work of Davisson and Germer, de Broglie’s hypothesis has been extensively tested with various experimental techniques, and the existence of de Broglie waves has been confirmed for numerous elementary particles. Neutrons have been used in scattering experiments to determine crystalline structures of solids from interference patterns formed by neutron matter waves. The neutron has zero charge and its mass is comparable with the mass of a positively charged proton. Both neutrons and protons can be seen as matter waves. Therefore, the property of being a matter wave is not specific to electrically charged particles but is true of all particles in motion. Matter waves of molecules as large as carbon \(C_{60}\) have been measured. All physical objects, small or large, have an associated matter wave as long as they remain in motion. The universal character of de Broglie matter waves is firmly established.

Example \(\PageIndex{3A}\): Neutron Scattering

Suppose that a neutron beam is used in a diffraction experiment on a typical crystalline solid. Estimate the kinetic energy of a neutron (in eV) in the neutron beam and compare it with kinetic energy of an ideal gas in equilibrium at room temperature.

We assume that a typical crystal spacing a is of the order of 1.0 Å. To observe a diffraction pattern on such a lattice, the neutron wavelength λ must be on the same order of magnitude as the lattice spacing. We use Equation \ref{6.61} to find the momentum p and kinetic energy K . To compare this energy with the energy \(E_T\) of ideal gas in equilibrium at room temperature \(T = 300 \, K\), we use the relation \(K = 3/2 k_BT\), where \(k_B = 8.62 \times 10^{-5}eV/K\) is the Boltzmann constant.

We evaluate pc to compare it with the neutron’s rest mass energy \(E_0 = 940 \, MeV\):

\[p = \frac{h}{\lambda} \Rightarrow pc = \frac{hc}{\lambda} = \frac{1.241 \times 10^{-6}eV \cdot m}{10^{-10}m} = 12.41 \, keV. \nonumber \]

We see that \(p^2c^2 << E_0^2\) and we can use the nonrelativistic kinetic energy:

\[K = \frac{p^2}{2m_n} = \frac{h^2}{2\lambda^2 m_n} = \frac{(6.63\times 10^{−34}J \cdot s)^2}{(2\times 10^{−20}m^2)(1.66 \times 10^{−27} kg)} = 1.32 \times 10^{−20} J = 82.7 \, meV. \nonumber \]

Kinetic energy of ideal gas in equilibrium at 300 K is:

\[K_T = \frac{3}{2}k_BT = \frac{3}{2} (8.62 \times 10^{-5}eV/K)(300 \, K) = 38.8 \, MeV. \nonumber \]

We see that these energies are of the same order of magnitude.

Neutrons with energies in this range, which is typical for an ideal gas at room temperature, are called “thermal neutrons.”

Example \(\PageIndex{3B}\): Wavelength of a Relativistic Proton

In a supercollider at CERN, protons can be accelerated to velocities of 0.75 c . What are their de Broglie wavelengths at this speed? What are their kinetic energies?

The rest mass energy of a proton is \(E_0 = m_0c^2 = (1.672 \times 10^{−27} kg)(2.998 \times 10^8m/s)^2 = 938 \, MeV\). When the proton’s velocity is known, we have β = 0.75 and \(\beta \gamma = 0.75 / \sqrt{1 - 0.75^2} = 1.714\). We obtain the wavelength λλ and kinetic energy K from relativistic relations.

\[\lambda = \frac{h}{p} = \frac{hc}{\beta \gamma E_0} = \frac{1.241 \, eV \cdot \mu m}{1.714 (938 \, MeV)} = 0.77 \, fm \nonumber \]

\[K = E_0(\gamma - 1) = 938 \, MeV (1 /\sqrt{1 - 0.75^2} - 1) = 480.1\, MeV \nonumber \]

Notice that because a proton is 1835 times more massive than an electron, if this experiment were performed with electrons, a simple rescaling of these results would give us the electron’s wavelength of (1835)0.77 fm = 1.4 pm and its kinetic energy of 480.1 MeV /1835 = 261.6 keV.

Exercise \(\PageIndex{3}\)

Find the de Broglie wavelength and kinetic energy of a free electron that travels at a speed of 0.75 c .

\(\lambda = 1.417 \, pm; \, K = 261.56 \, keV\)

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Class 12 Physics (India)

Course: class 12 physics (india)   >   unit 11, de broglie wavelength.

• Comparing de Broglie wavelengths: Solved example
• de Broglie wavelength

Want to join the conversation?

• Upvote Button navigates to signup page
• Downvote Button navigates to signup page
• Flag Button navigates to signup page

Video transcript

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

18.2: Quantized Energy - De Broglie's Hypothesis and the Schroedinger Equation

• Last updated
• Save as PDF
• Page ID 151775

• Paul Ellgen
• Oklahoma School of Science Mathematics

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Subsequent to Planck’s proposal that energy is quantized, the introduction of two further concepts led to the theory of quantum mechanics. The first was Einstein’s relativity theory, and his deduction from it of the equivalence of matter and energy. The relativistic energy of a particle is given by

\[E^2=p^2c^2+m^2_0c^4 \nonumber \]

where \(p\) is the momentum and \(m_0\) is the mass of the particle when it is at rest. The second was de Broglie’s hypothesis that any particle of mass \(m\) moving at velocity \(v\), behaves like a wave. De Broglie’s hypothesis is an independent postulate about the structure of nature. In this respect, its status is the same as that of Newton’s laws or the laws of thermodynamics. Nonetheless, we can construct a line of thought that is probably similar to de Broglie’s, recognizing that these are heuristic arguments and not logical deductions.

We can suppose that de Broglie’s thinking went something as follows: Planck and Einstein have proposed that electromagnetic radiation—a wave-like phenomenon—has the particle-like property that it comes in discrete lumps (photons). This means that things we think of as waves can behave like particles. Conversely, the lump-like photons behave like waves. Is it possible that other lump-like things can behave like waves? In particular is it possible that material particles might have wave-like properties? If a material particle behaves like a wave, what wave-like properties should it exhibit?

Well, if we are going to call something a wave, it must have a wavelength, \(\lambda\), a frequency, \(\nu\), and a propagation velocity, \(v\), and these must be related by the equation \(v=\lambda \nu\). The velocity of propagation of light is conventionally given the symbol \(c\), so \(c=\lambda \nu\). The Planck-Einstein hypothesis says that the energy of a particle (photon) is \(E=h\nu ={hc}/{\lambda }\). Einstein proposes that the energy of a particle is given by \(E^2=p^2c^2+m^2_0c^4\). A photon travels at the speed of light. This is compatible with other relativistic equations only if the rest mass of a photon is zero. Therefore, for a photon, we must have \(E=pc\). Equating these energy equations, we find that the momentum of a photon is

\[p={h}/{\lambda } \nonumber \]

Now in a further exercise of imagination, we can suppose that this equation applies also to any mass moving with any velocity. Then we can replace \(p\) with \(mv\), and write \[mv={h}/{\lambda } \nonumber \]

We interpret this to mean that any mass, \(m\), moving with velocity, \(v\), has a wavelength, \(\lambda\), given by

\[\lambda ={h}/{mv} \nonumber \]

This is de Broglie’s hypothesis . We have imagined that de Broglie found it by a series of imaginative—and not entirely logical—guesses and suppositions. The illogical parts are the reason we call the result a hypothesis rather than a derivation, and the originality of the guesses and suppositions is the reason de Broglie’s hypothesis was new. It is important physics, because it turns out to be experimentally valid. Very small particles do exhibit wave-like properties, and de Broglie’s hypothesis correctly predicts their wavelengths.

In a similar vein, we can imagine that Schrödinger followed a line of thought something like this: de Broglie proposes that any moving particle behaves like a wave whose wavelength depends on its mass and velocity. If a particle behaves as a wave, it should have another wave property; it should have an amplitude. In general, the amplitude of a wave depends on location and time, but we are thinking about a rather particular kind of wave, a wave that—so to speak—stays where we put it. That is, our wave is supposed to describe a particle, and particles do not dissipate themselves in all directions like the waves we get when we throw a rock in a pond. We call a wave that stays put a standing wave; it is distinguished by the fact that its amplitude depends on location but not on time.

Mathematically, the amplitude of any wave can be described as a sum of (possibly many) sine and cosine terms. A single sine term describes a simple wave. If it is a standing wave, its amplitude depends only on distance, and its amplitude is the same for any two points separated by one wavelength. Letting the amplitude be \(\psi\), this standing wave is described by \(\psi \left(x\right)=A{\mathrm{sin} \left(ax\right)\ }\), where \(x\) is the location, expressed as a distance from the origin at \(x=0\). In this wave equation, \(A\) and \(a\) are parameters that fix the maximum amplitude and the wavelength, respectively. Requiring the wavelength to be \(\lambda\) means that \(a\lambda =2\pi\). (Since \(\psi\) is a sine function, it repeats every time its argument increases by \(2\pi\) radians. We require that \(\psi\) repeat every time its argument increases by \(a\lambda\) radians, which requires that \(a\lambda =2\pi\).) Therefore, we have \[a={2\pi }/{\lambda } \nonumber \]

and the wave equation must be

\[\psi \left(x\right)=A{\mathrm{sin} \left({2\pi x}/{\lambda }\right)\ } \nonumber \]

Equations, \(\psi\), that describe standing waves satisfy the differential equation

\[\frac{d^2\psi }{dx^2}=-C\psi \nonumber \]

where \(C\) is a constant. In the present instance, we see that

\[\frac{d^2\psi }{dx^2}=-{\left(\frac{2\pi }{\lambda }\right)}^2A{\mathrm{sin} \left(\frac{2\pi x}{\lambda }\right)\ }=-{\left(\frac{2\pi }{\lambda }\right)}^2\psi \nonumber \]

From de Broglie’s hypothesis, we have \(\lambda ={h}/{mv}\), so that the constant \(C\) can be written as

\[C={\left(\frac{2\pi }{\lambda }\right)}^2={\left(\frac{2\pi mv}{h}\right)}^2={\left(\frac{2\pi }{h}\right)}^2\left(2m\right)\left(\frac{{mv}^2}{2}\right)=\left(\frac{8{\pi }^2m}{h^2}\right)\left(\frac{{mv}^2}{2}\right) \nonumber \]

Let \(T\) be the kinetic energy, \({{mv}^2}/{2}\), and let \(V\) be the potential energy of our wave-like particle. Then its energy is \(E=T+V\), and we have \({{mv}^2}/{2}=T=E-V\).

The constant \(C\) becomes

\[C=\left(\frac{8{\pi }^2m}{h^2}\right)T=\left(\frac{8{\pi }^2m}{h^2}\right)\left(E-V\right) \nonumber \]

Making this substitution for \(C\), we find a differential equation that describes a standing wave, whose wavelength satisfies the de Broglie equation. This is the time-independent Schrödinger equation in one dimension:

\[\frac{d^2\psi }{dx^2}=-\left(\frac{8{\pi }^2m}{h^2}\right)\left(E-V\right)\psi \nonumber \] or \[-\left(\frac{h^2}{8{\pi }^2m}\right)\frac{d^2\psi }{dx^2}+V\psi =E\psi \nonumber \]

Often the latter equation is written as

\[\left[-\left(\frac{h^2}{8{\pi }^2m}\right)\frac{d^2}{dx^2}+V\right]\psi =E\psi \nonumber \]

where the expression in square brackets is called the Hamiltonian operator and abbreviated to \(H\), so that the Schrödinger equation becomes simply, if cryptically,

\[H\psi =E\psi \nonumber \]

If we know how the potential energy of a particle, \(V\), depends on its location, we can write down the Hamiltonian operator and the Schrödinger equation that describe the wave properties of the particle. Then we need to find the wave equations that satisfy this differential equation. This can be difficult even when the Schrödinger equation involves only one particle. When we write the Schrödinger equation for a system containing multiple particles that interact with one another, as for example an atom containing two or more electrons, analytical solutions become unattainable; only approximate solutions are possible. Fortunately, a great deal can be done with approximate solutions.

The Schrödinger equation identifies the value of the wavefunction, \(\psi \left(x\right)\), with the amplitude of the particle wave at the location x. Unfortunately, there is no physical interpretation for \(\psi \left(x\right)\); that is, no measurable quantity corresponds to the value of \(\psi \left(x\right)\). There is, however, a physical interpretation for the product \(\psi \left(x\right)\psi \left(x\right)\) or \({\psi }^2\left(x\right)\). [More accurately, the product \(\psi \left(x\right){\psi }^*\left(x\right)\), where \({\psi }^*\left(x\right)\) is the complex conjugate of \(\psi \left(x\right)\). In general, \(x\) is a complex variable.] \({\psi }^2\left(x\right)\) is the probability density function for the particle whose wavefunction is \(\psi \left(x\right)\). That is, the product \({\psi }^2\left(x\right)dx\) is the probability of finding the particle within a small distance, \(dx\), of the location \(x\). Since the particle must be somewhere, we also have

\[1=\int^{+\infty }_{-\infty }{{\psi }^2\left(x\right)}dx \nonumber \]

• Modern Physics
• De Brogile Waves
• Active page

Modern Physics of Class 12

De broglie waves.

The first step in the understanding of the hidden meaning of Bohr's quantum orbits was made by a Frenchman, Louis de Broglie, who tried to draw an analogy between the sets of discrete energy levels that characterise the inner state of atoms and the discrete sets of mechanical vibrations that are observed in the case of violin strings, organ pipes etc.

de Broglie asked himself, 

"Could it not be that the optical properties of atoms are due to some kind of standing waves enclosed within themselves?"

As a result of these considerations, de Broglie came out with his hypothesis that the motion of electron within the atom is associated with a peculiar kind of waves which he called "pilot waves".

In order to have n complete wavelengths (nλ) fit into the circumference of the nth orbit, the following relation must be true:

nλn = 2πrn

From Bohr's theory of the hydrogen atom,

The wavelength of the wave associated with a moving particle is equal to Planck's quantum constant divided by the momentum of the particle.

Example  16.6

What is the energy and wavelength of a thermal neutron ? 

By definition, a thermal neutron is a free neutron in a neutron gas at about 20o C (293 K).

Since it has three degrees of freedom, therefore 

Example 16.7

A particle of mass m is confined to a narrow tube of length L. 

(a) Find the wavelengths of the de-Broglie waves which will resonate in the tube,

(b) Calculate the corresponding particle momenta, and 

(c) Calculate the corresponding energies.

  (a) The de Broglie waves will resonate with a node at each end of the tube.

A few of the possible resonance forms are as follows : 

λn = 2L/n ;  n = 1, 2, 3, ......

• Structure Of Atoms
• Radioactivity
• Atomic Nucleus
• Photoelectric Effect
• solved question

Talk to Our counsellor

• Rotational Motion

Angular Momentum Of Electron

In this article, we will be learning about the angular momentum of electrons in detail. We will also be learning about De Broglie’s Explanation of the Quantization of Angular Momentum of Electrons.

What is Angular Momentum of Electron?

Bohr’s atomic model laid down various postulates for the arrangement of electrons in different orbits around the nucleus. According to Bohr’s atomic model, the angular momentum of electrons orbiting around the nucleus is quantized. He further added that electrons move only in those orbits where the angular momentum of an electron is an integral multiple of h/2. This postulate regarding the quantisation of angular momentum of an electron was later explained by Louis de Broglie. According to him, a moving electron in its circular orbit behaves like a particle-wave.

The angular momentum of an electron by Bohr is given by mvr or nh/2π (where v is the velocity, n is the orbit in which the electron is revolving, m is mass of the electron, and r is the radius of the nth orbit).

Among the various proposed models over the years, the Quantum Mechanical Model seems to best fit all properties. Watch the video to learn more about the features of the quantum mechanical model.

De Broglie’s Explanation to the Quantization of Anfgular Momentum of Electron:

The behaviour of particle waves can be viewed analogously to the waves travelling on a string. Particle waves can lead to standing waves held under resonant conditions. When a stationary string is plucked, a number of wavelengths are excited. On the other hand, we know that only those wavelengths survive which form a standing wave in the string, that is, which have nodes at the ends.  

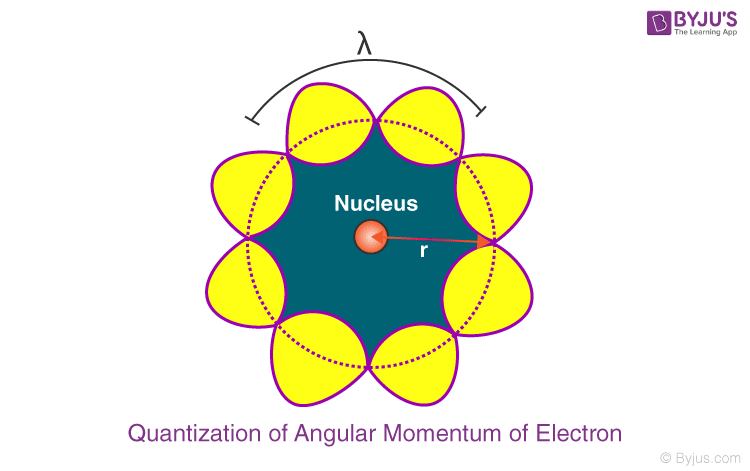
Thus, in a string, standing waves are formed only when the total distance travelled by a wave is an integral number of wavelengths. Hence, for any electron moving in k th circular orbit of radius r k , the total distance is equal to the circumference of the orbit, 2πr k .

Let this be equation (1).

λ is the de Broglie wavelength.

We know that de Broglie wavelength is given by:

p is electron’s momentum

h = Planck’s constant

Let this be equation (2).

Where mv k is the momentum of an electron revolving in the k th orbit. Inserting the value of λ from equation (2) in equation (1) we get,

Hence, de Broglie hypothesis successfully proves Bohr’s second postulate stating the quantization of angular momentum of the orbiting electron. We can also conclude that the quantized electron orbits and energy states are due to the wave nature of the electron.

Frequently Asked Questions – FAQs

Is it possible for electrons to have angular momentum, how to find the angular momentum of an electron, is the angular momentum of an electron quantised, what is bohr’s atomic model, who proposed the quantization of angular momentum.

To learn more physics concepts with the help of interactive and engaging video lessons, download BYJU’S – The Learning App.

Put your understanding of this concept to test by answering a few MCQs. Click ‘Start Quiz’ to begin!

Select the correct answer and click on the “Finish” button Check your score and answers at the end of the quiz

Visit BYJU’S for all Physics related queries and study materials

Your result is as below

Request OTP on Voice Call

Leave a Comment Cancel reply

Your Mobile number and Email id will not be published. Required fields are marked *

Post My Comment

Register with BYJU'S & Download Free PDFs

Register with byju's & watch live videos.